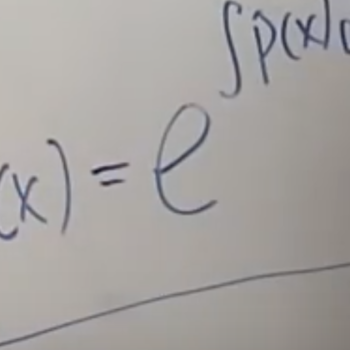Question

How do you use substitution to integrate `4 / ((3x + 6)^2) dx`?

Answer 1

use the u substitution

`u=3x+6`

`du=3dx`

to get `4` multiply it by `4/3`

`4/3*du=4/cancel(3)*cancel(3)dx`

`4/3du=4dx`

bring the integral into the u world

`int(4/3)/u^2`

bring the constant into the front

`4/3int1/u^2`

use the index law

`1/y^z=y^-z`

`4/3intu^-2`

use the power rule for integration and integrate

`intx^n=x^(n+1)/(n+1)`

`=4/3intu^-2`

`4/3(u^(-2+1)/(-2+1))+C`

`4/3(u^(-1)/(-1))+C`

bring it into the `x` world

`4/3(-(3x+6)^-1)+C`

`4/3(-1/(3x+6))+C`

finally multiply it

`-4/(9x+18)+C`