How do you use the discriminant to determine the numbers of solutions of the quadratic equation #x^2 + 6x - 7 = 0# and whether the solutions are real or complex?

1 Answer
Tony B
Feb 5, 2016

see explanantion

Explanation:

Consider the following value for the discriminant: (d)

#d>0 ->#The plot crosses the x-axis so has 2 solutions

#d=0->#The plot is such that it does not cross the x-axis but the#" "# axis forms a tangent to the max/min

#d<0->#The plot does not cross nor come into contact with the #" "#x-axis. Thus any solution to the expression being #" "#equated to zero will result in a complex number solution.

For your equation of: #x^2+6x-7=0#
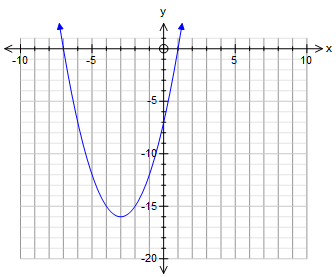
Tony B

The discriminant is:

#sqrt(b^2-4ac) -> sqrt(6^2-4(1)(-7))#

#sqrt(6^2+28)" ">" "0" " =>" " 2" solutions"#

As these are not complex they are real solutions

Related questions
See all questions in Solutions Using the Discriminant
Impact of this question
1708 views around the world
You can reuse this answer
Creative Commons License