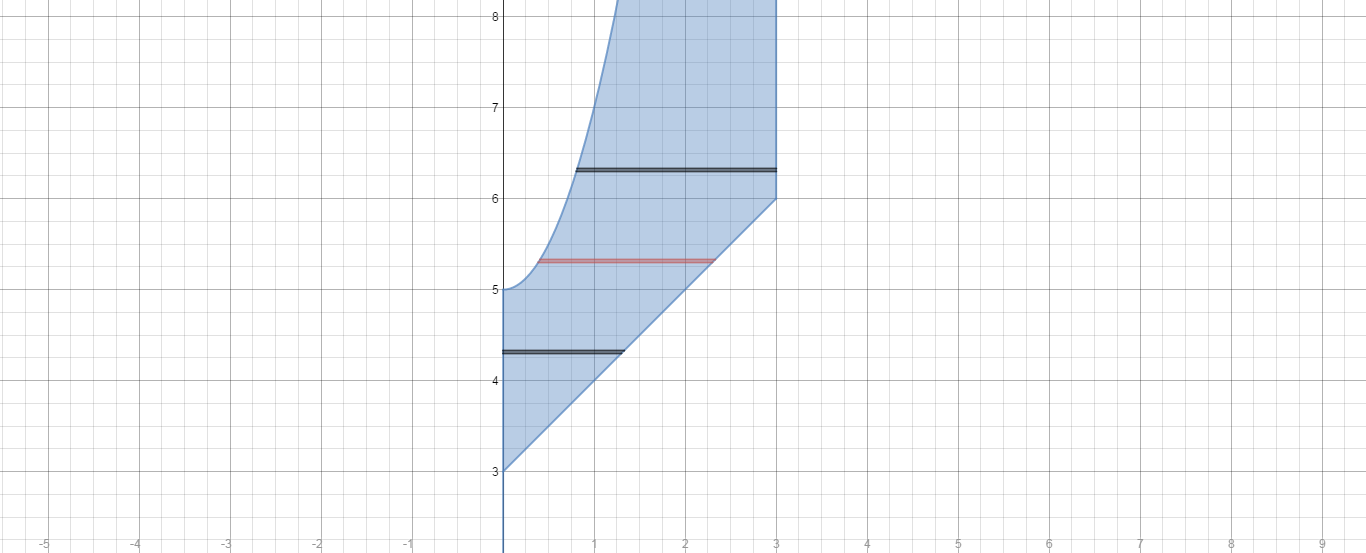
Here is a graph of part of the region to be rotated about the #x# axis.
In order to use shells, we must take our representative slice parallel to the axis of rotation. In this case, that's the #x# axis, so the thickness of our shells will be #dy#.
That means we'll need to rewrite the curves as functions of #y#
#x=sqrt((y-5)/2)#, #x=y-3#
Every shell will have #"radius" =y#, #"thickness"=dy# and #"height" = x_"right" - x_"left"#
As the graph shows, there are three separate integrals we need to do, because the calculation of #x_"right"# and #x_"left"# change as #y# goes from #3# (the lower bound) to #23# (the uppr bound, not on my graph).
(Are we sure we want to use shells for this?)
From #y=3# to #y=5# , the height of the cylindrical shell will be
#"height" = x_"right" - x_"left" = (y-3)-0 = y-3#
So we need to integrate
#int_3^5 2piy(y-3)dy = 2pi int_3^5 (y^2-3y)dy# which is left to the student.
From #y=5# to #y=6#, the height of the cylindrical shell will be
#"height" = x_"right" - x_"left" = (y-3)- sqrt((y-5)/2)#
So we need to integrate
#int_5^6 2piy(y-3- sqrt((y-5)/2))dy = 2pi int_5^6 (y^2-3y- ysqrt((y-5)/2))dy# the last term of which can be integrated by substitution (or parts).
From #y=6# to #y=23#, the height of the cylindrical shell will be
#"height" = x_"right" - x_"left" = 3- sqrt((y-5)/2)#
So we need to integrate
#int_6^23 2piy(3- sqrt((y-5)/2))dy = 2pi int_6^23 (3y- ysqrt((y-5)/2))dy# the last term of which can be integrated by substitution (or parts).
Washers
To use washers take the slices perpendicular to the axis of rotation.
As x varies from #0# to #3#, the greater radius at #x# is #R = 2x^2+5# and the lesser radius is #r = x+3#,
We need to integrate
#int_0^3 pi (R^2-r^2)dx = pi int_0^3 ((2x^2+5)^2-(x+3)^2)dx# which is simply the integral of a degree 4 polynomial when we expand it.


