Question

How many electrons can have the quantum number set n=3 and ml=-2?

Answer 1

Two electrons.

Explanation:

As you know, four quantum numbers are used to describe the position and spin of an electron that's part of a given atom, with each electron being described by an unique set of quantum numbers.

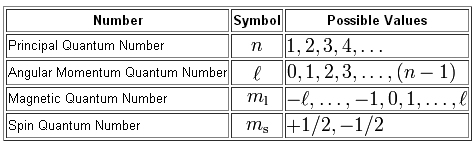
In your case, the problem provides with the values of the principal quantum number, `n`, and of the magnetic quantum number, `m_l`.

More specifically, the problem provides you with the energy level on which the electron resides, and the specific orbital in which it is located.

Your job now will be to determine how many subshell can hold that specific orbital.

Notice that the value of the magnetic quantum number depends on the value of the angular momentum quantum number, `l`, the one that describes the subshell in which you can find the electron.

In order to have `m_l = -2`, you need to have `l>=2`, since `m_l` takes values ranging from `-l` to `l`.

Since you need to have `0 < l < n`, it follows that the only possible value you can have for `l` will be

`l = 2 ->` the d-subshell

Since each orbital can hold a maximum of two electrons, the number of electrons that can share the two quantum number `n=3` and `m_l = -2` will be equal to `2`, each having opposite spin.

`n = 3, l=2, m_l = -2, m_s = -1/2`

This set describes an electron located on the third energy level, in the 3d-subshell, in the `3d_(z^2)` orbital, and having spin down.

`n = 3, l = 2, m_l = -2, m_s = +1/2`

This set describes an electron located on the third energy level, in the 3d-subshell, in the `3d_(z^2)` orbital, and having spin up.