How to solve this equation? #2cosx+1=0;x in[0,3pi)#;

Question

4068 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-04-05T17:28:20

#(2pi)/3, (4pi)/3, (8pi)/3#

#2cosx+1=0 #

#cosx= -1/2 #

Now we know from this handy "CAST" nmemonic that cos is negative in Q2+Q3:

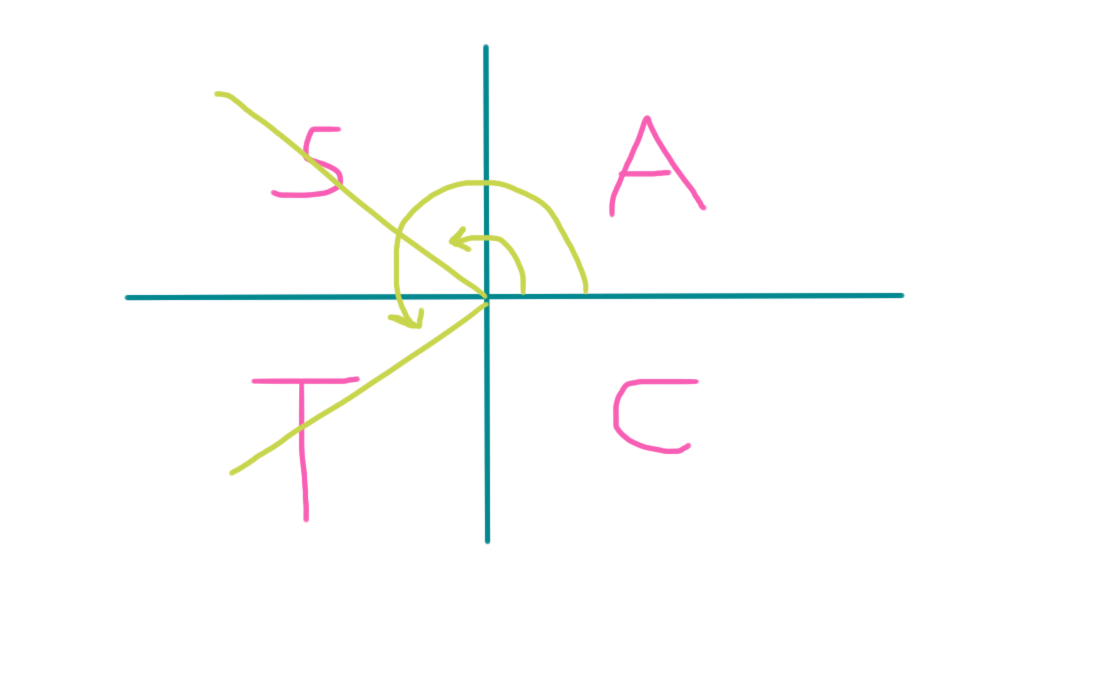
Rembrandt

We can even draw the triangles:

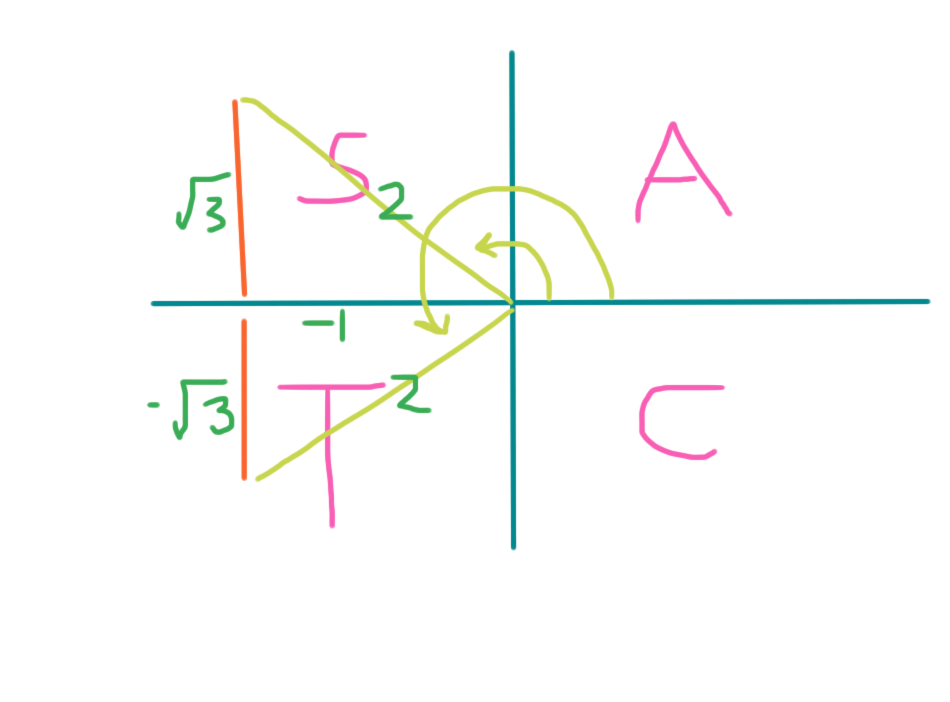
Picasso

So in Q1: #cos x = 1/2 implies x = pi/3#

The corresponding angle in Q2 is #pi - pi/3 = (2pi)/3#

The corresponding angle in Q3 is #pi + pi/3 = (4pi)/3#

Now because it is #x in[0,3pi)#, we are not done yet.

After spinning round a full #2 pi#, we have another #pi# to go which takes us back into Q2.

The second corresponding angle in Q2 is #2 pi + (pi - pi/3) = (8pi)/3#