In the given figure initially spring is in its natural length and system is released from rest. Finally block again comes to rest at mean position due to air resistance. Find the total work done by?
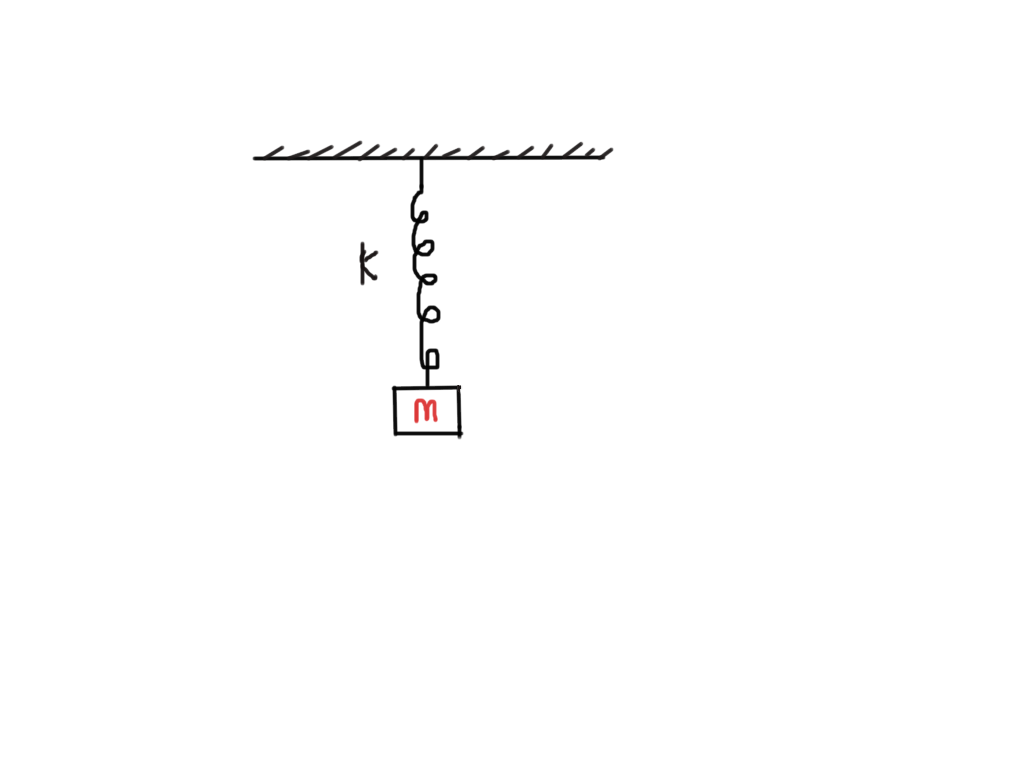
1) gravitational force
2) spring force
3) force due to air resistance

1) gravitational force
2) spring force
3) force due to air resistance
2 Answers
This is what I get
Explanation:
When the system is released from rest, the mass
#F_G=Mg#
where#g# is acceleration due to gravity.
This force acts on the spring (assumed mass-less) which initially is in natural unstreched position. Let the elongation of the spring be
#Mg=ky_0#
#=>y_0=(Mg)/k# .....(1)
At the mean position of the system, taken as origin of coordinates, let mass have velocity
- Initial total energy equation of the system
#E_i=PE_"spring"+GPE_"Mass"+KE_"Mass"#
#=>E_i=0+Mgy_0+0#
#=>E_i=Mgy_0# - Final total energy equation of the system
#E_f=PE_"spring"+GPE_"Mass"+KE_"Mass"+E_"air friction"#
#=>E_f=1/2ky_0^2+0+0+E_"air friction"#
#=>E_f=1/2ky_0^2+E_"air friction"#
Using Law of conservation of energy we get
#Mgy_0=1/2ky_0^2+E_"air friction"#
#=>E_"air friction"=Mgy_0-1/2ky_0^2#
Work done by
- gravitational force
#=Mgy_0# - spring force
#=1/2ky_0^2# - force due to air resistance
#=Mgy_0-1/2ky_0^2#
where#y_0# is given by (1).
Definition of work:
Assuming this question is a reference to an (under) damped oscillation, it is helpful for a moment to ignore the damping (air resistance, friction, whatever dissipative force is at play).
-
Over an undamped oscillation, gravity will do zero new work on the suspended mass. Gravity is
#bbF_g = mg \ bbhat y# , it always acts in the downward positive y-direction, so it variously encourages and opposes motion ie does positive or negative work. -
Over half an undamped oscillation, the spring will do zero new work on the suspended mass. The spring is restorative, always acting toward the centre of the oscillation:
#bbF_s = - k y \ bb hat y# . Again the spring variously encourages and opposes motion ie does positive or negative work on the suspended mass.
Adding the damping will not change this. It is the damping that extracts the energy from the mass-spring system. The physics is simple. As the mass collides with the air molecules, its energy is dissipated. The mass does work on its surroundings, or if you like, the air resistance does negative work on the mass-spring.
With a judiciously chosen damping term, and positive y downwards:
#implies m ddot y + k y + m nu dot y - m g = 0 qquad triangle#
The total energy
Which from
Solving

