Prove that the magnetic moment of a hydrogen atom in its ground state is eh/(4πm)?
Current, i= #e/t#
i= #e omega/(2pi)#
angular momentum, L= #(nh)/(2pi)# = mvr
Current, i=
i=
angular momentum, L=
1 Answer
Consider an electron in the ground state revolving around a proton in a circular orbit of radius
We know that
#v=romega#
where#omega# is the angular velocity
Current flowing due motion of electron is equal to the charge flowing through any point per second which can be found with the help of time period
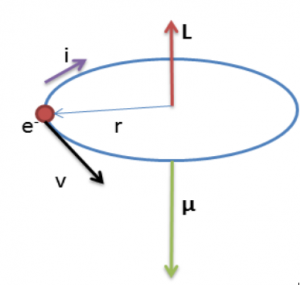
The magnetic moment
#mu = iA#
Referring to the figure above, magnetic moment of the electron is
#mu = (−ev)/(2πr) pir^2#
#=>mu= (−erv)/2# ..........(2)
We know that angular momentum of the electron is
# vecL= vecrxx(m_evecv)#
Since radial and velocity vector are orthogonal
#:.L=rm_ev# .......(3)
Also
#L = (nh)/(2π)#
For ground state principal quantum number
#:.L=(h)/(2π)# ......(4)
Using (3) to eliminate
#mu= (−e(L/m_e))/2#
#=>mu= (−e)/(2m_e)L# ......(5)
Negative sign shows that angular momentum and magnetic moment vectors have opposite directions as shown in the figure.
Using (4) above equation can be written as
#mu= (−e)/(2m_e)((h)/(2π))#
#mu= (−eh)/(4pim_e)#
