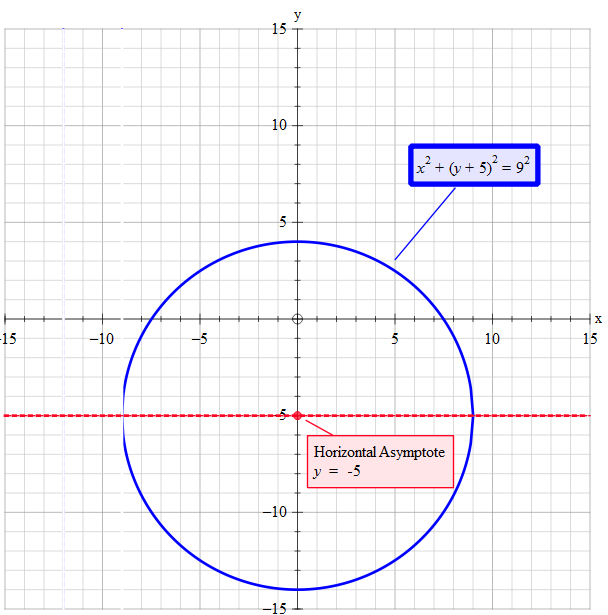
The equation of a circle is #x^2+(y+5)^2=81#. What is the center and radius of the circle?
2 Answers
Explanation:
The equation of a circle is given by
where
We have
We can rewrite this as
Centre
Radius
Explanation:
Proper word for 'moving' is 'transpose'.
Suppose you had some function of
Then this will form a particular shape depending on what the form of
If we changed this to
On the other hand
So for the x-axis:
a plus 'shifts' left
a minus 'shifts' right.
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Similarly for y-axis:
a plus 'shifts' down
a minus 'shifts' up