What is the distance between the following polar coordinates?: # (2,(pi)/2), (2,(17pi)/12) #
1 Answer
Jan 1, 2018
Explanation:
Graph the two points:
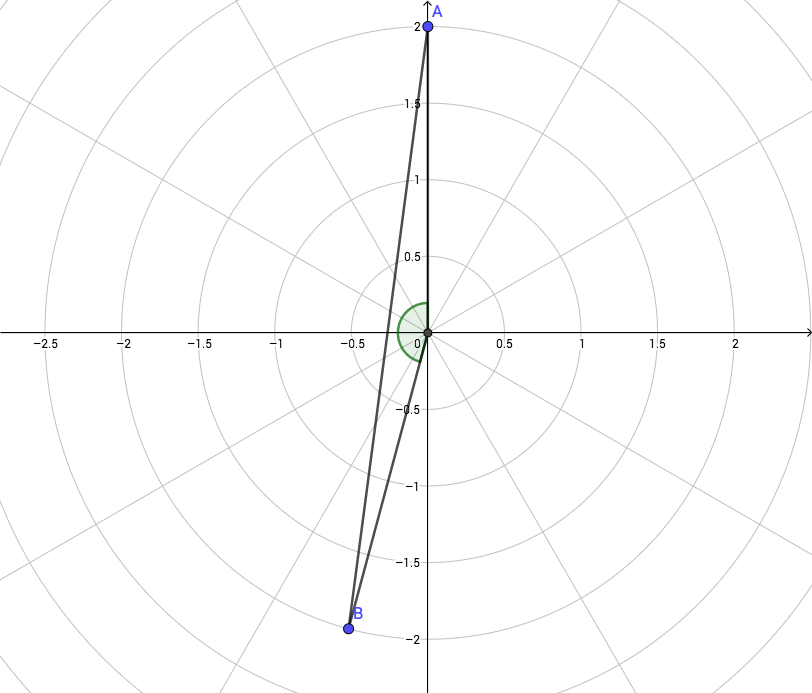
A triangle is formed when the two points
Then,

