Question

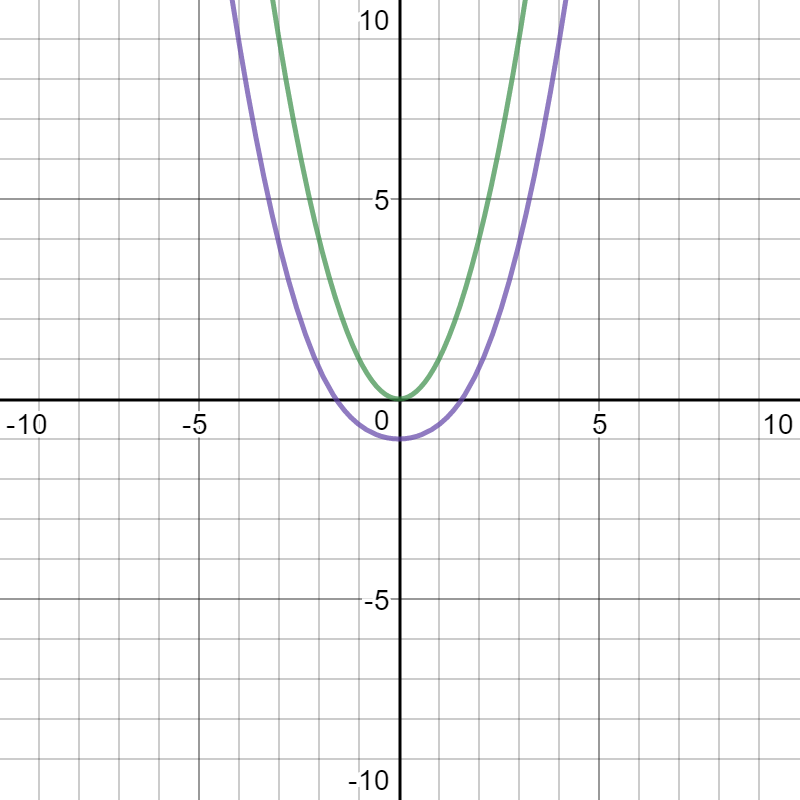
What's the curve parallel to the parabola `y=x^2`?

Answer 1

The parametric equations are
`(x,y) = (1/(2a)t- ((k)t)/sqrt(1-t^2), 1/(4a)t^2-k/sqrt(1-t^2))`

Where k is the distance from `y = ax^2`

Explanation:

I found a good answer in a forum

I have written the parametric equations into the answer area.

Here is a graph of `y = x^2` and its parallel curve with `k = -1`:

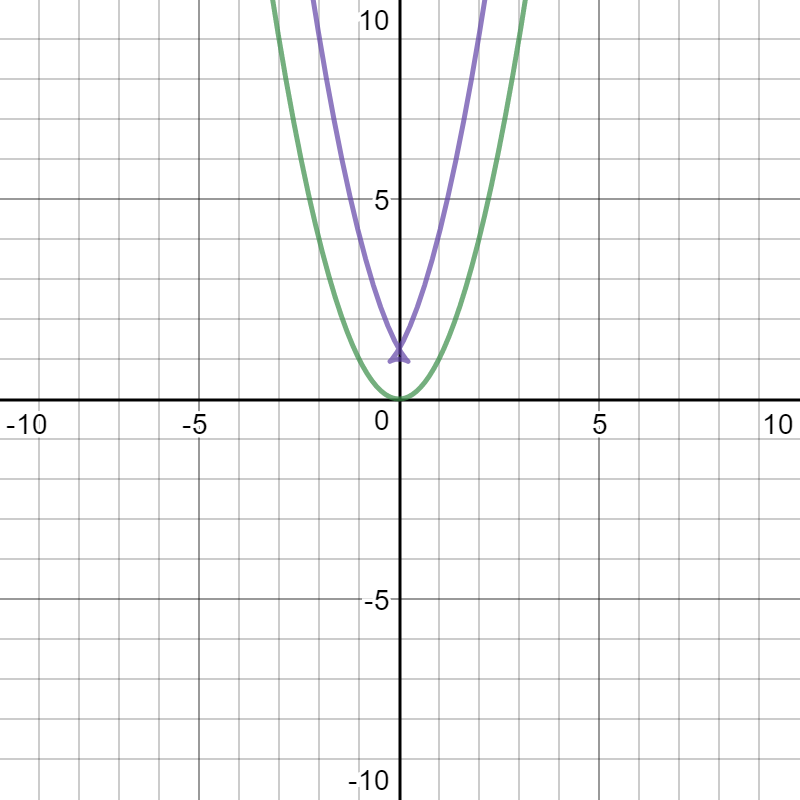
Here is a graph of `y = x^2` and its parallel curve with `k = 1`: