Question

Which equation best represents the line perpendicular to 2y=7x if the y-intercept is b=5?

Answer 1

`y=-2/7x+5`

Explanation:

`2y=7x` , rewrite as:

`y=7/2x`
The slope is `7/2`, the slope of perpendicular lines are negative reciprocal, thus the slope is`-2/7`, then the equation of the line is:

`y=-2/7x+5`

Answer 2

`y = -2/7x+5`

Explanation:

First find the slope of the given line which is

`2y = 7x`

solve for `y`

`y = 7/2x`

here the coefficient of x is the slope

the slope is =`7/2`

now the line that we need to find is perpendicular so its slope is
the reciprocal of `7/2` with a different sign so the slope of our line is =`-2/7`

the equation of the line that is perpendicular to `2y=7x` is

`y = -2/7x+5`

+5 because is given in the question that it will intersect y-axis at 5

---

you can check you equation by graphing them using graph calculators like

https://www.desmos.com/calculator

Desmos is a great calculator to graph equations and functions

Answer 3

The line perpendicular to `2y=7x` is `z=-2/7x+5`

Explanation:

We should know that if you have a line `y=ax+b`, then `z=-1/ax+c` is perpendicular to `y`, like in the following graph, where
`y=-1/2x-1` is perpendicular to `y=2x+3`.

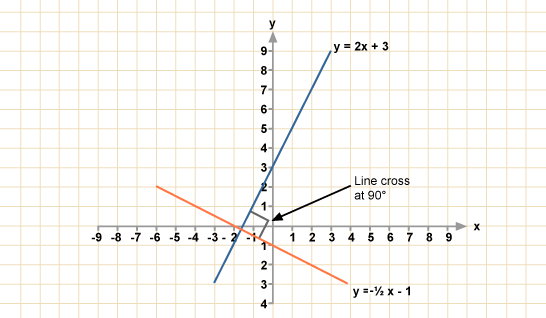
As this is a well known fact, I will take it as given. (See for instance http://www.bbc.co.uk/schools/gcsebitesize/maths/algebra/graphshirev1.shtml or http://www.coolmath.com/algebra/08-lines/14-perpendicular-lines-01

So let's write our line on the above form:

`2y=7x => y=7/2x`
This means `a=7/2`, so we get `z=-2/7x+5` since the perpendicular should go through `(0, 5)`.

Graphically we get: