Question

How do you graph `y=x^2-2x+3`?

Answer 1

You can look at the "special" points of your function. These are points that characterize the curve represented by your function.
In your case you have a quadratic in the general form given as:
`y=ax^2+bx+c`
which is represented, graphically, by a PARABOLA. The orientation of the parabola is given by the coefficient `a` of `x^2`; in this case you have `a=1>0` so this is an upward parabola, i.e. like a U.

Now the special points:
1) You find the VERTEX (the lowest point of your parabola) which has coordinates given by:
`x_v=-b/(2a) and y_v=-Delta/(4a)`;
(Where `Delta=b^2-4ac`);
2) Y-axis intecept: The coordinates of this pont are given as: `(c,0)`;
3) X-axis intercept(s): the coordinate of these intercepts (if they exist) are given by putting `y=0` and solving the second degree equation:
`ax^2+bx+c=0`

In your case you have:

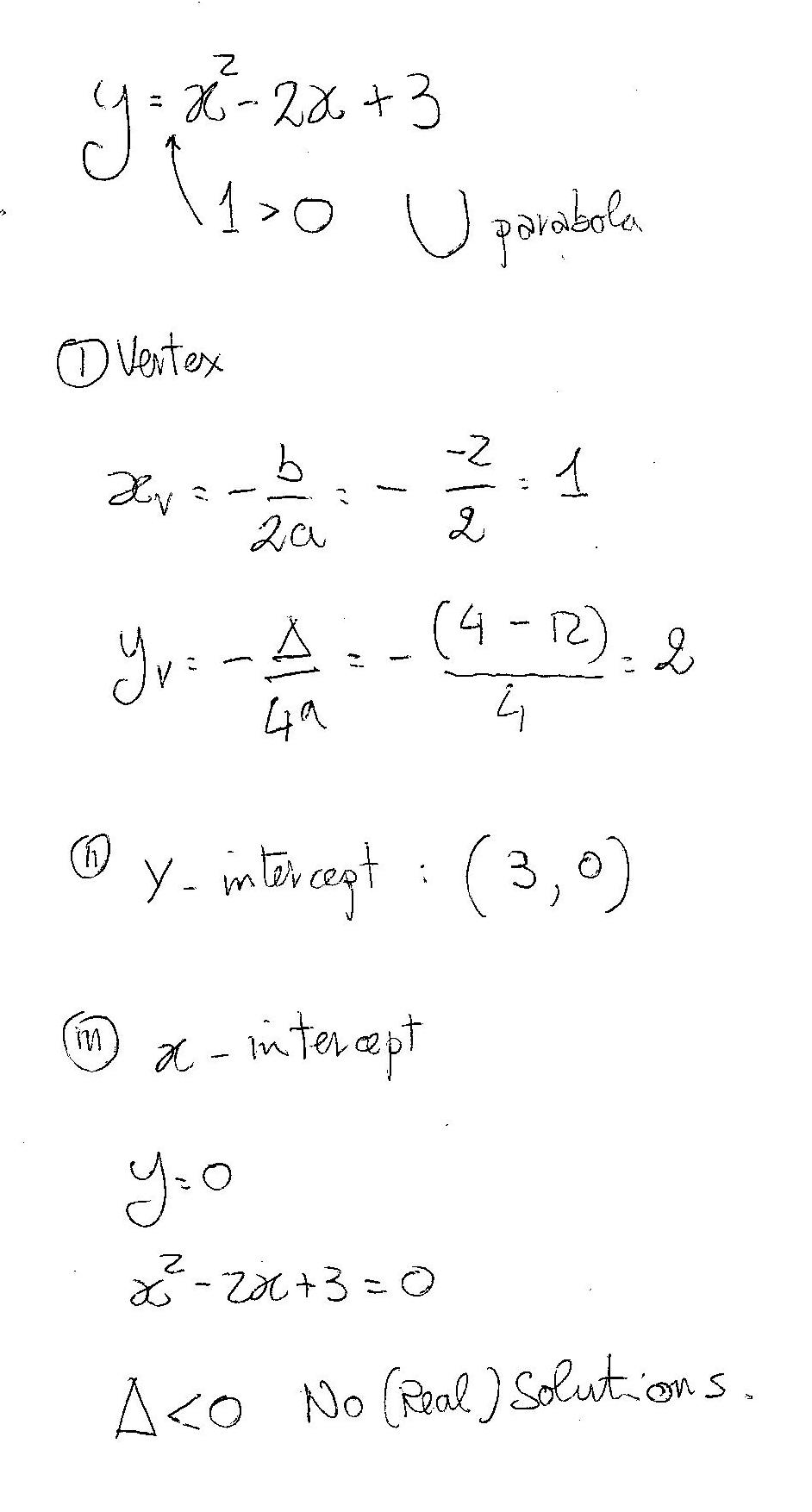
With only these points we can already plot our parabola: