Question

Question #ebbe0

Answer 1

The mass of the collected hydrogen gas is `"0.0648 g"`.

Once again, here's the reaction wou're working with

`Ca_((s)) + 2H_2O_((l)) -> Ca(OH)_2(aq) + H_2(g)`

The basic idea behind this reaction is that the hydrogen gas will be collected over water at a total pressure that includes the water vapor.

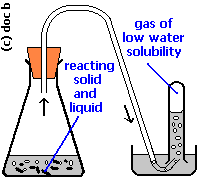
So, in order to determine the pressure of the hydrogen gas, you must remove the water vapor from the total pressure

`P_("total") = P_("hydrogen") + P_("water") => P_("hydrogen") = P_("total") - P_("water")`

In this case,

`P_("hydrogen") = "988 mmHg" - "31.82 mmHg" = "956.18 mmHg"`

Now just use the ideal gas law equation to solve for the moles of hydrogen produced

`PV = nRT => n = (PV)/(RT)`

`n = (956.18/760"atm" * 641 * 10^(-3)"L")/(0.082 ("atm" * "L")/("mol" * "K") * (273.15 + 30)"K") = "0.0324 moles hydrogen"`

Once again, use the units required for this value of R - atm, L, and K!

Now just use hydrogen's molar mass to determine the actual mass

`"0.0324 moles" * ("2.0 g")/("1 mole") = "0.0648 g"` `H_2`