How do you combine #(5x+2)/(x-4) + (x+3)/(x+1)#?

Question

1694 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2015-05-20T23:55:54

Try this:
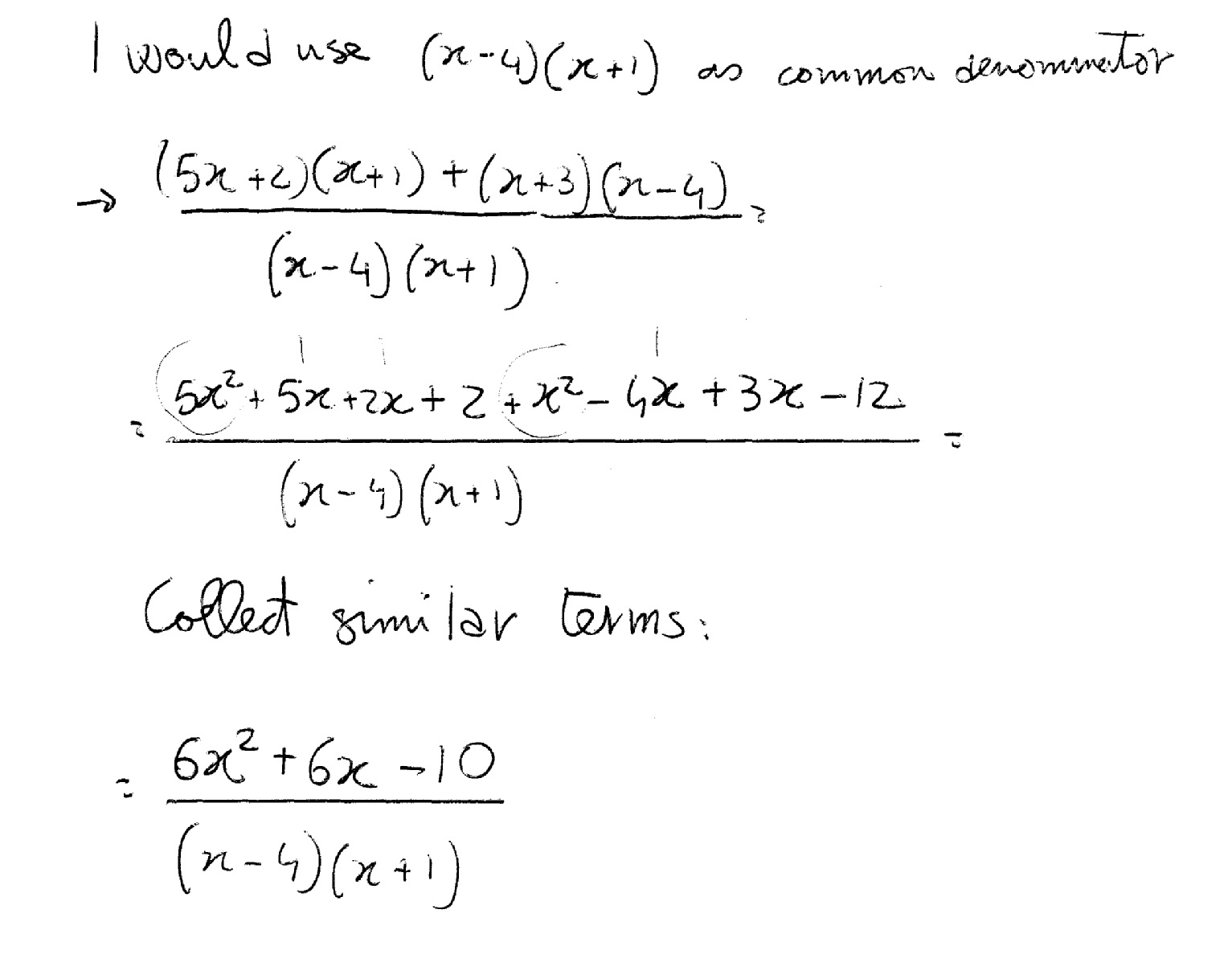
enter image source here

Answer 2 · 2015-05-21T00:21:23

The answer is #(2(3x^2+3x-5))/((x+1)(x-4))#.

Sorry, this is a long answer.

Problem: Combine #(5x+2)/(x-4)+(x+3)/(x+1)# .

Multiply the numerator and denominator of #(5x+2)/(x-4)# times #(x+1)#.

#((5x+2)(x+1))/((x+1)(x-4))#

Multiply the numerator and denominator of #(x+3)/(x+1)# times #(x-4)#.

#((x+3)(x-4))/((x+1)(x-4))#

We now have common denominators. Combine terms.

#((5x+2)(x+1)+(x+3)(x-4))/((x+1)(x-4))# =

#(5x^2+5x+2x+2+x^2-4x+3x-12)/((x+1)(x-4)# =

Group like terms.

#(5x^2+x^2+5x+2x-4x+3x+2-12)/((x+1)(x-4))# =

Simplify.

#(6x^2+6x-10)/((x+1)(x-4))#

Factor out the GCF #2#.

#(2(3x^2+3x-5))/((x+1)(x-4))#