Question

Which set of quantum numbers is allowed?

1) `(n,l,m_l,m_s) = (6,3,-3,+1/2)`
2) `(n,l,m_l,m_s) = (2,2,1,+1/2)`
3) `(n,l,m_l,m_s) = (2,1,-1,-1/2)`
4) `(n,l,m_l,m_s) = (4,2,1,0)`
5) `(n,l,m_l,m_s) = (2,1,-1/2,+1/2)`
6) `(n,l,m_l,m_s) = (0,1,-1,-1/2)`

Answer 1

The correct sets are (1) and (3).

You can think of quantum numbers as being coordinates used to describe the position and spin of an electron in an atom.

There are four quantum numbers, each with its own set of acceptable values

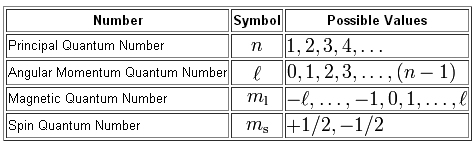
The spin quantum number, `m_s`, is the only on that's independent of the value of `n`, the principal quantum number, and can only take two possible values, +1/2, which means spin-up, and -1/2, which means spin-down.

The angular momentum quantum number, `l`, depends on the value of `n`, while the magnetic quantum number, `m_l`, depends on the value of `l`.

This being said, let's start with the given sets

• Set (1)

All the quantum numbers have allowed values. This particular set describes an electron located in the 6th energy level, in one of the seven 6f-orbitals, and having spin-up.

• Set (2)

Notice that this set has `"n=2"` and `"l=2"`. Since `l` cannot be equal to `n`, this set is not allowed, as it can't correctly describe the position of an electron.

• Set (3)

Once again, all the quantum numbers have allowed values. This set describes an electron located in the 2nd energy level, in the `2p_y` orbital, and having spin-down.

• Set (4)

This set is not allowed because `m_s"=0"`. Since the spin quantum numbercan only by +1/2 or -1/2, this set cannot correctly describe the position of an electron.

• Set (5)

This time, the value of the magnetic quantum number is not allowed. Notice that `m_l"=-1/2"`, which falls outside of the allowed values of `-l, ..., 0, ... , +l`.

• Set (6)

This set is not allowed because `"n=0"` is not an allowed value for the principal quantum number.