Question

How do you find all the asymptotes for function `y=(2x^2 + 5x- 3)/(3x+1)`?

Answer 1

See the explanation.

Explanation:

The line `x=-1/3` is a vertical asymptote because the denominator, but not the numerator is `0` at `-1/3`

There is no horizontal asymptote, because as `x` increases [decreases] without bound, `y` also increases [decreases] without bound.

If "all the asymptotes" includes oblique asymptotes, then do the division to get:

`(2x^2+5x-3)/(3x+1) = 2/3x+13/9-(40/9)/(3x+1)`

So

`y=2/3x+13/9` is an oblique asymptote.

Answer 2

Vertical Asymptote: `x=-1/3`
Slant Asymptote: `y=2/3x+13/9`.

Explanation:

Vertical Asymptote
To get the vertical asymptote, find the value of `x` that will make the denominator `0` (undefined).

`3x+1=0`
`3x=-1`
`x=-1/3`

The vertical asymptote is `color(blue)(x=-1/3)`.

Horizontal/Slant Asymptote
Since the degree of the numerator is greater than the degree of the denominator by one, we will get a slant asymptote. To solve this, divide `2x^2+5x-3` by `3x+1` using long division. The answer will be your slant asymptote.

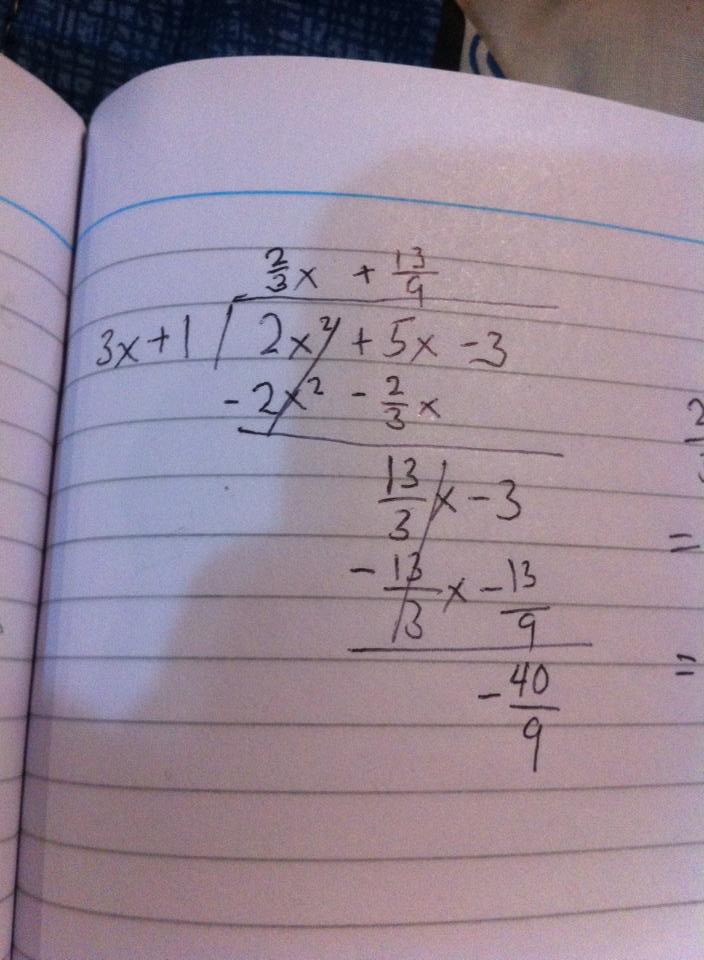(Sorry for my handwriting)

Never mind the remainder. The only thing you have to take note of is the polynomial on top.

The slant asymptote is `color(red)(y=2/3x+13/9)`.