How do you find the volume of the solid generated by revolving the region bounded by the curves x=y-y^2 rotated about the y-axis?
1 Answer
Oct 26, 2015
See the explanation section below.
Explanation:
Here is a picture of the region with a representative slice taken perpendicular to the axis of rotation. This is a set-up to use disks to find the volume. The thickness is
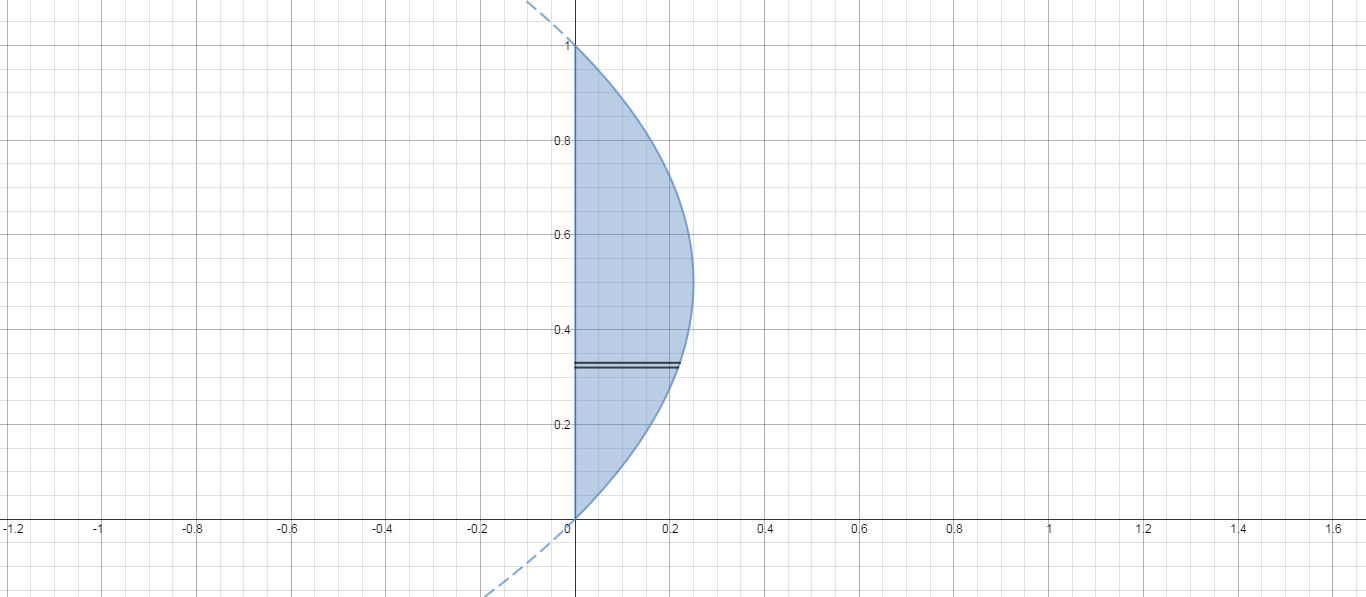
As
The volume of the representative disk is
So, to find the volume of the solid, we need to evaluate
This integral evaluates to
Bonus
Here is a link to the same volume problem using shells instead of disks.

