How do you graph (x-2)^2 + (y+5)^2?
1 Answer
Oct 28, 2015
Transposed circle.
Explanation:
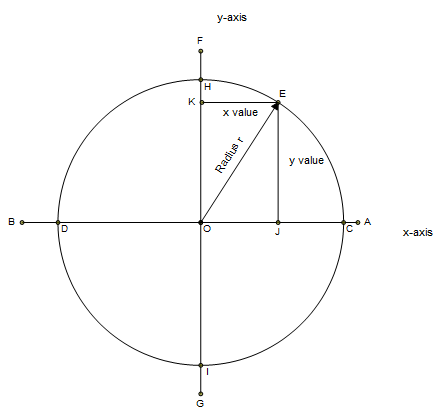
This is the equation of a circle so you should really have stated it as:
This equation is derived from Pythagoras relating the sides of a triangle where
Changing the values of x and y by constant values means that you have moved the centre of the circle away from the origin. The origin being
So the centre has moved to -2 on the x-axis and to +5 on the y axis.
To actually draw the graph you need to know the value of the radius
Circle at the origin. Move every point to
So put the needle of your compass at