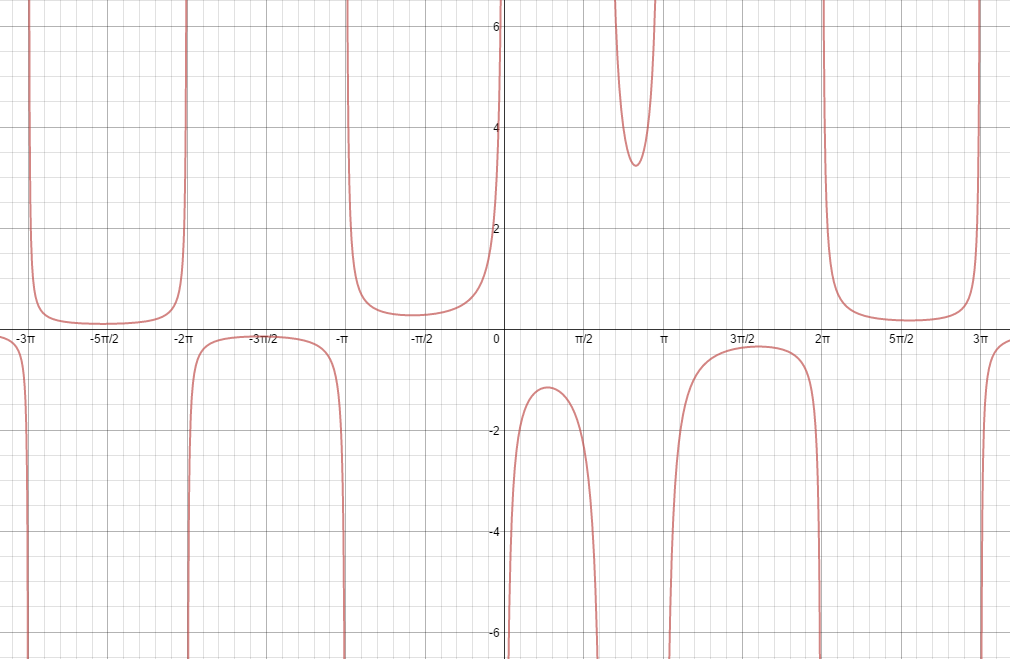
For what values of x, if any, does $f (x) = \frac{1}{(x - 2) sin x}$ $f(x) = 1/((x-2)sinx)$ have vertical asymptotes?

Question

For what values of x, if any, does $f (x) = \frac{1}{(x - 2) sin x}$ $f(x) = 1/((x-2)sinx)$ have vertical asymptotes?

1 Answer

Impact of this question

1801 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-03-13T05:14:40

$x = 2$ $x=2$ , $x = 2 π k$ $x=2pik$ , and $x = π + 2 π k$ $x=pi+2pik$ where $k \in Z$ $k in Z$

Explanation:

Vertical asymptotes occur whenever the denominator equals 0. To find them, we simply set the denominator to 0 and solve, like thus:
$(x - 2) sin x = 0$ $(x-2)sinx=0$
$x - 2 = 0$ $x-2=0$ and $sin x = 0$ $sinx=0$
$x = 2$ $x=2$ and $x = 2 π k, π + 2 π k$ $x=2pik, pi+2pik$ where $k \in Z$ $k in Z$ ( $k$ $k$ is an integer)

We have infinitely many vertical asymptotes, at $x = 2$ $x=2$ , $x = 2 π k$ $x=2pik$ , and $x = π + 2 π k$ $x=pi + 2pik$ . We can see this on the graph of the function below:
enter image source here

Science

Math

Humanities

... and beyond

For what values of x, if any, does $f (x) = \frac{1}{(x - 2) sin x}$ $f(x) = 1/((x-2)sinx)$ have vertical asymptotes?

1 Answer

Explanation:

Related questions

Impact of this question

Science

Math

Humanities

... and beyond

For what values of x, if any, does f(x)=1(x−2)sinxf(x) = 1/((x-2)sinx) have vertical asymptotes?

1 Answer

Explanation:

Related questions

Impact of this question

For what values of x, if any, does $f (x) = \frac{1}{(x - 2) sin x}$ $f(x) = 1/((x-2)sinx)$ have vertical asymptotes?