Question

How do you integrate `int 1/sqrt(-e^(2x) +9)dx` using trigonometric substitution?

Answer 1

`int(1/sqrt(-e^(2x)+9))=(ln(sqrt(9-e^(2x))-3)-x)/3`

Explanation:

Here is the corresponding right triangle from which you can extract all the parts for substitution:

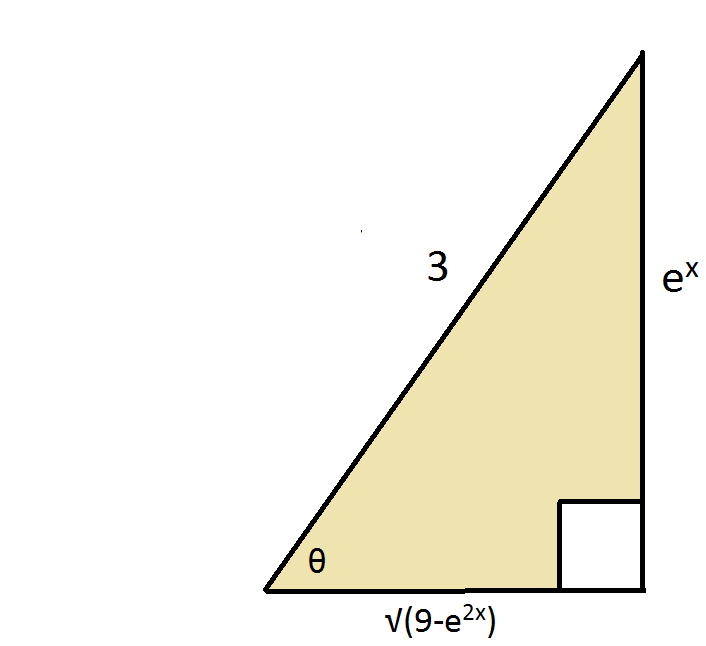
You need to find the following and then systematically replace all x terms (rectangular) with `theta` terms (trig):

x

`dx/(d theta)`

`sqrt(9-e^(2x))`

I'll start you off in the right direction:

Since

#sin(theta) =e^x/3 #

We have then that:

`x = ln(3*sin(theta))`

Now differentiate with respect to `theta` and split up the differentials.

You can then rewrite that entire integrand in terms of `theta` and solve using trigonometric substitution.

I'll give you one more piece and then I gotta sleep!

What is `cos(theta)?`

Well, referencing the triangle you can see that:

`cos (theta) = sqrt(9-e^(2x))/3`

This says that:

`sqrt(9-e^(2x)) =3*cos(theta)`

You'll still need to find `d theta`

So now you can systematically replace all rectangular terms with
trigonometric terms.

That's your job!