How do you find the slant asymptote of #f(x) = (x^2 + 6x - 9)/(x - 2)#?
1 Answer
Explanation:
The asymptotes are a tendency that occur at particular point. In this case these points will be
As
However. If this is investigated further it soon becomes evident that this is only a very rough estimate and is slightly out.
If you actually carry out a polynomial division you end up with:
As x increases in magnitude then
So you end up with the asymptote being
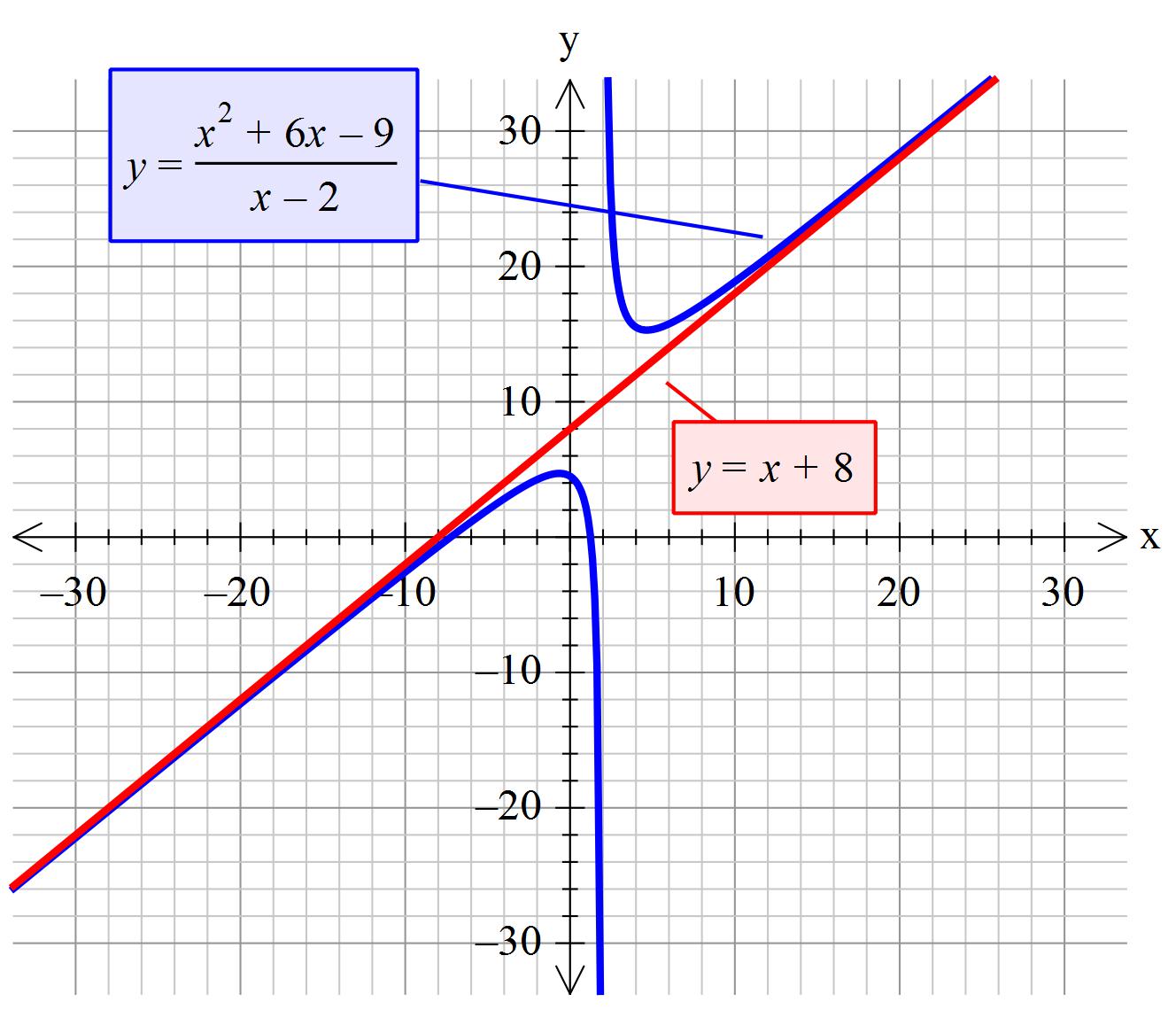
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~
They do not ask for the vertical asymptote. So just for the sake of interest. You are not allowed to divide by 0. Mathematically this is given the name of being 'undefined'
So the denominator becomes 0 at
So 2 is the 'excluded value'

