Question

How do you find the value of the discriminant and determine the nature of the roots `8b^2-6b+3=5b^2`?

Answer 1

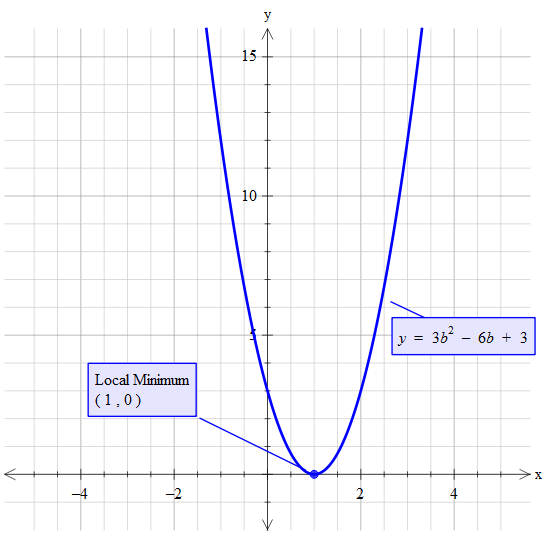
The nature of the 'roots' is that 'they' both have the same value. Some people refer to this as duplicity.

In effect, there is only one root.

Explanation:

Given:`" "8b^2-6b+3=5b^2`

Subtract `5b^2` from both sides

`3b^2-6b+3=0`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

To avoid confusion let `b=t`

Then we have: `3t^2-6t+3`

Compare to `y=at^2+bt+c=0`

`a=3"; "b=-6"; "c=3`

Where `t=(-b+-sqrt(b^2-4ac))/(2a)`

`=>t=(+6+-sqrt(6^2-4(3)(3)))/(2(3))`

The discriminant is:

`b^2-4ac ->36 - 36 = 0`

When the discriminant is 0 it means that the x-axis is tangential to the curve at the maximum/minimum point.

The there is a single value solution.