How do you solve #6cos^2x+5cosx+1=0# in the interval [0,360]?
1 Answer
Explanation:
Let's take a look at the equation:
I find it hard to focus on factoring when looking at trig functions within a polynomial, so let's just get rid of that for a second by saying:
Now let's factor:
And so therefore:
And so:
Ok - so now what?
Cosine is negative where the adjacent is negative (that is to say, where the line along the x-axis making the triangle we're looking at runs along the negative part of the axis). So we're in quadrants 2 and 3.
Let's work out the reference angles, starting with
This one is a special triangle - the 30/60/90 triangle - and so we know the reference angle is
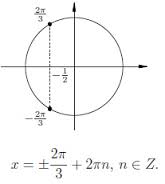
And now
This one isn't so neat. It's roughly

