Question

Suppose you know that 3 is a zero of the function `g(x) = 4x^3-x^2-27x -18` What must be a factor of the polynomial in g(x)?

Answer 1

Technically, `x - 3`, since by the remainder theorem, a zero of a function will be a number, that when inserted in the function, will give a remainder of `0`. If you're looking for another zero of the function, we will have to divide `4x^3 - x^2 - 27x - 18` by `x- 3`.

By synthetic division:

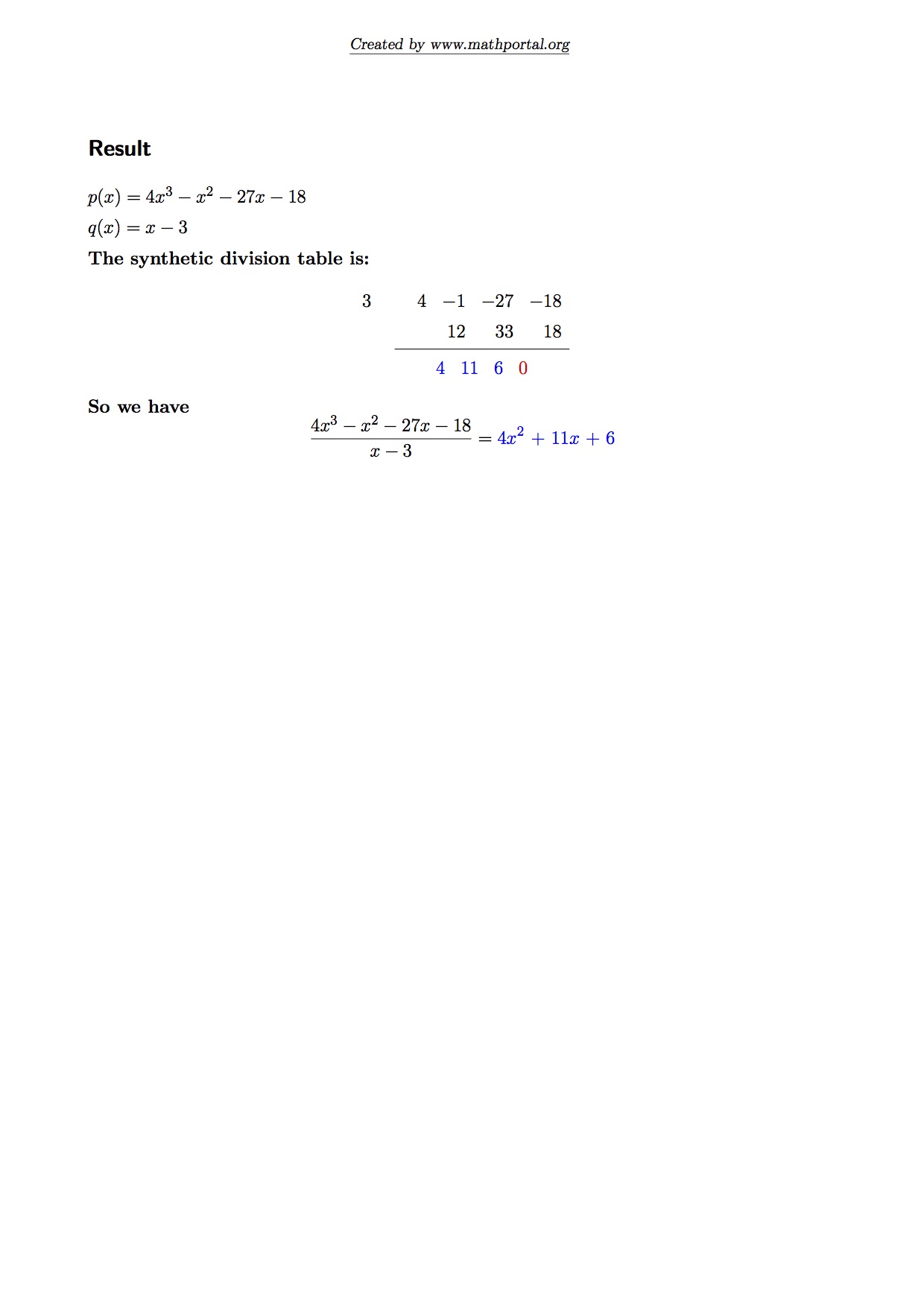
So, the quotient is `4x^2 + 11x + 6`. This can be factored as follows.

`=4x^2 + 8x + 3x + 6`

`=4x(x + 2) + 3(x + 2)`

`= (4x + 3)(x + 2)`

Hence, two other factors are `x + 2` and `4x + 3`.

Hopefully this helps!