Kinetic Energy Question?

Question

Car A with a mass of 1000kg is travelling east at 25m/s when it collides with car B, 2000kg, travelling north at 15m/s. After the collision, the two cars stick together and have the same velocity. Assume that they slide without friction after collision.

(a) What is the final velocity of the combined mass of cars (magnitude and direction)?

(b) How much kinetic energy was lost in this process and where did it go?

3553 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-11-28T11:09:37

Hi, have a look and try to evaluate it...I cannot finish it now!

have a look and try evaluate $v_x$ and $v_y$ ....you can then use trigonometry and Pythagoras to characterize $v$ ::

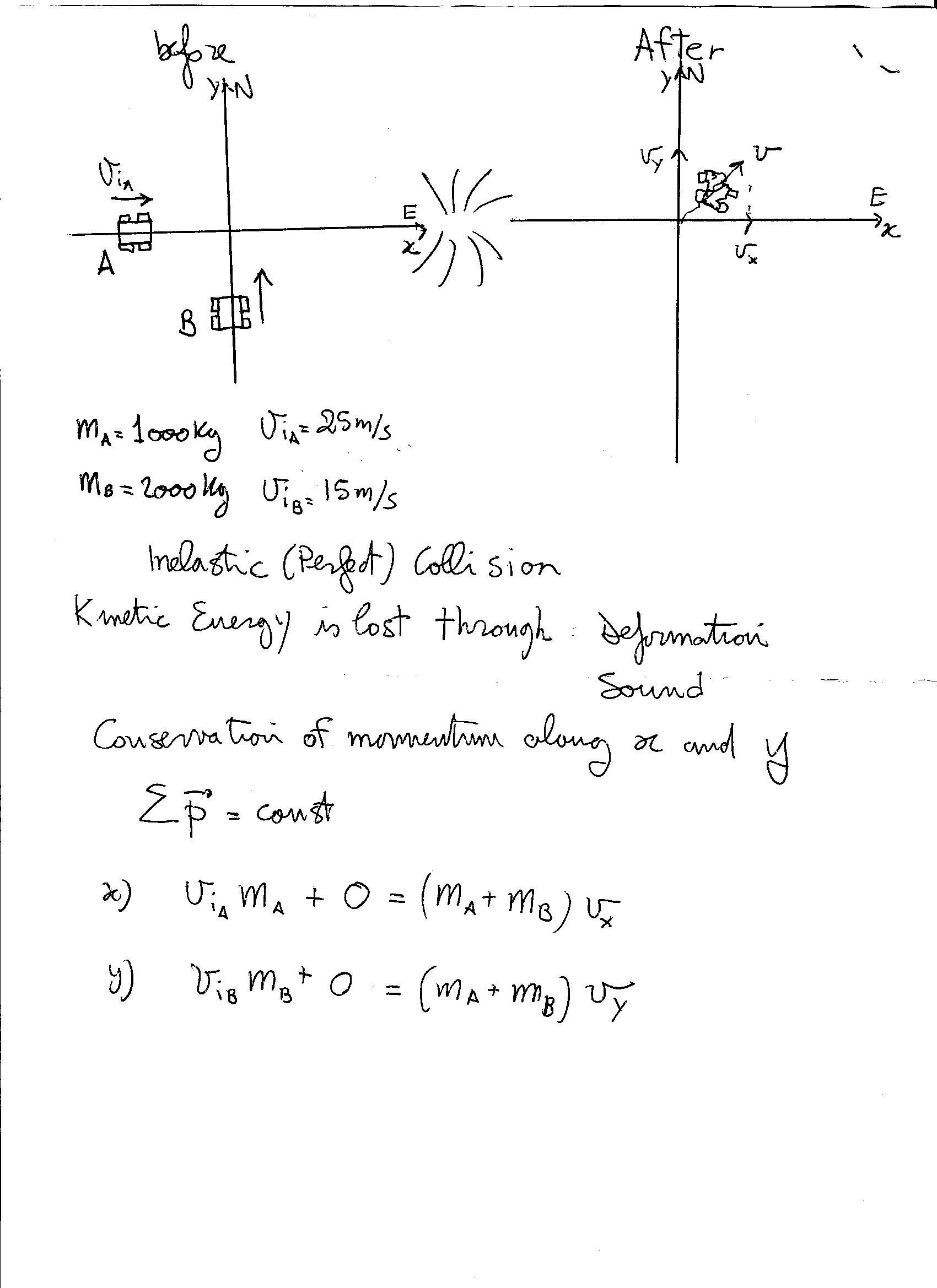
enter image source here

Answer 2 · 2016-11-28T13:01:17

See below.

In the absence of external forces

$Delta vec p=0$ so

$m_(b_1)vec v_(b_1)+m_(b_2)vec v_(b_2) = m_(a_1)vec v_(a_1)+m_(a_2)vec v_(a_2)$

Here we have

$m_(b_1)=1000, vec v_(b_1)=25 hati$
$m_(b_2)=2000, vec v_(b_2)=15 hatj$

$m_(a_1)=1000, vec v_(a_1)= vec v_a$
$m_(a_2)=2000, vec v_(a_2)= vec v_a$

so

$1000 cdot 25 hati+2000 cdot 15 hatj = (1000+2000)vec v_a$

so

$vec v_a=(25/3 hat i+10 hat j)$

with

$abs (vec v_a)=(5 sqrt[61])/3 = 13.02$

$angle vec v_a = arctan(10//(25/3)) = 50.2^@$

Kinetic energy before $K_b=1/2m_(b_1) abs(vec v_(b_1))^2+1/2m_(b_2) abs(vec v_(b_2))^2$

Kinetic energy after

$1/2(m_(b_1)+m_(b_2))abs(vec v_a)^2$

so

$Delta K = K_a-K_b =-283333.$ [J]