Question

Which of the following set of quantum numbers is not allowed?

A) `n=1, l=0, m_l=0, m_s=-1/2`
B) `n=3,l=2, m_l=-1, m_s=1/2`
C) `n=5, l=2, m_l=-3, m_s=-1/2`
D) `n=4, l=3, m_l=-2, m_s=1/2`

Answer 1

The answer is C).

Explanation:

All you have to do here is to use the definitions of the four quantum numbers that we use to describe the location and spin of an electron inside an atom.

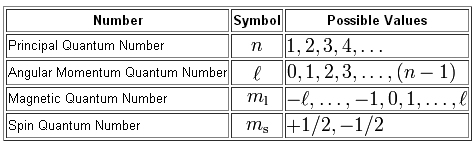
Notice that the magnetic quantum number, `m_l`, depends on the value of the angular momentum quantum number, `l`, as described by

`m_l = {-l, -(l+1), ..., -1, 0 ,1, ..., (l+1), l}`

This tells you that the absolute value of the magnetic quantum number cannot exceed the value of the angular momentum quantum number

`|m_l| <= l`

For option C), you have `l+2` and `m_l = -3`. This cannot take place because

`|-3| color(red)(cancel(color(black)( <=))) 2`

In other words, the `d` subshell, which is described by `l=2`, can only hold a total of `5` orbitals, since

`m_l = { -2, -1, 0, 1, 2} ->` for the d subshell

Therefore, you can say that

`n=5, l = 2, m_l = -3, m_s = -1/2`

is not a valid set of quantum numbers.

The other three sets of quantum numbers are indeed valid.

`n=1, l=0, m_l = 0, m_s = -1/2`

Describes an electron located on the first energy level, in the s subshell, in the `1s` orbital, that has spin-down

`n=3, l=2, m_l = -1, m_s = 1/2`

Describes an electron located on the third energy level, in the d subshell, in one of the five `3d` orbitals, that has spin-up

`n=4, l=3, m_l = -2, m_s = 1/2`

Describes an electron located on the fourth energy level, in the f subshell, in one of the seven `4f` orbitals, that has spin-up