Question

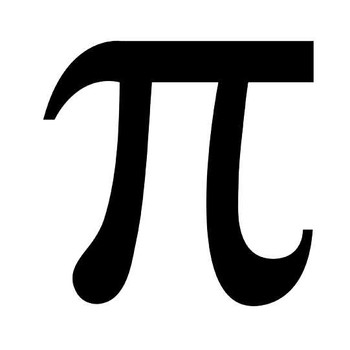
Find the maximum and minimum values for the function `f` defined by `f(x) = 2sinx + cos2x` in the interval `[0, pi/2]`?

Answer 1

Minimum:
`f(x) = {1, x=0, pi/2}`
Maximum:
`f(x) = {3/2, x=pi/6}`

Explanation:

`f(x) = 2sinx + cos2x`

`f'(x) = 2cosx - 2sin2x`

Now to find the critical number,

`f'(x) = 0`

`2cosx - 2sin2x = 0`
`2cosx-2(2sinxcosx) = 0`
`2cosx - 4sinxcosx = 0`
`2cosx(1-2sinx) = 0`

`2cosx=0`
`x = pi/2`

and `1-2sinx=0`
`x=pi/6`

Therefore,

When `x=0`, `f(x) = 2.0+1 = 1` and
when `x=pi/2, f(x) = 2.1-1=1`

And

when `x=pi/6, f(x) = 2.1/2+1/2 = 3/2`

Hence,

Minimum:
`f(x) = {1, x=0, pi/2}`
Maximum:
`f(x) = {3/2, x=pi/6}`