Question

What is the distance between the following polar coordinates?: `(5,(-15pi)/12), (5,(-7pi)/8)`

Answer 1

`5.56` (2dp)

Explanation:

Let `A=(5,(-15pi)/12)`, and `B=(5,(-7pi)/8)`

We can plot the polar coordinates as follows:

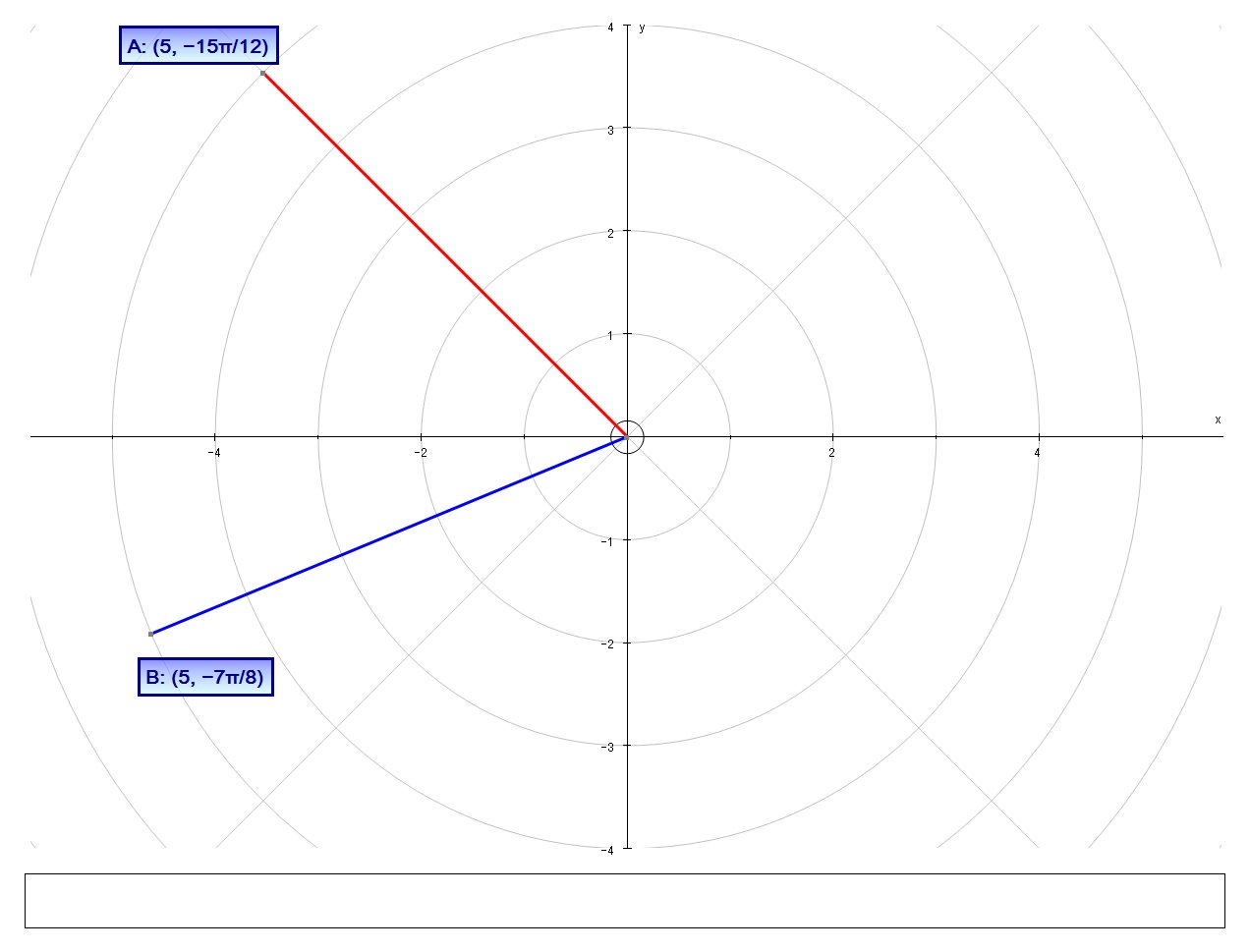
The angle between `A` and `B` is therefore given by:

`angle AOB = (-7pi)/8 - (-15pi)/12 = 3/8pi`

By the cosine rule we have:

`AB^2 = OA^2 + OB^2 - 2(OA)(OB)cos(angle AOB)`
`" " = 5^2 + 5^2 - 2(5)(5)cos(3/8pi)`
`" " = 25 + 25 - 50cos(3/8pi)`
`" " = 50 - 50cos(3/8pi)`
`" " = 30.865828 ...`

`:. AB = 5.555702 ...`