How do you find the solution to #sintheta+3=5sintheta# if #0<=theta<2pi#?

Question

2791 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-07-14T14:05:43

# theta = 0.848^r, 2.294^r #

We want to solve:

# sin theta + 3 = 5sin theta # where #theta in [0,2pi)#

We can write this as:

# \ \ 4sin theta = 3 #
# :. sin theta = 3/4 #

If we consider the graph of #y=sinx# then we can see there will be two solutions:

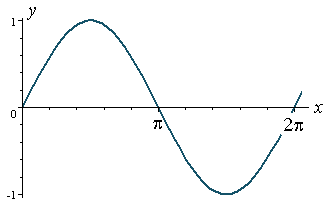
# sin theta = 3/4 => #
# " " theta_1 = arcsin(3/4)# (the fundamental value)
# " " \ \ \ = 0.848^r# (3dp)

So the two solutions in the specified range are:

# theta = theta_1, pi-theta_1 #
# \ \ = 0.848^r, 2.294^r #