Effective atomic number of #Fe# in #Fe_"2"(CO)_"9"# is ??
1 Answer
It would be
You can also find a worked effective atomic number example here.
The effective atomic number corresponds to the total number of electrons around the transition metal given by the ligands, PLUS the total number of electrons the transition metal brings.
Two methods are common to find the electrons donated by the ligands, which I will call:
- the Donor Pair method (Method A)
- the Neutral Ligand method (Method B)
THE DONOR PAIR METHOD
This method considers the ligand to donate a lone pair of electrons, and assigns the charges and atomic oxidation states accordingly. So,
THE NEUTRAL LIGAND METHOD
This method considers the ligand to give as many electrons for the bond as it would have if it were neutral. So,
The following table lists the number of donated electrons considered using both methods:
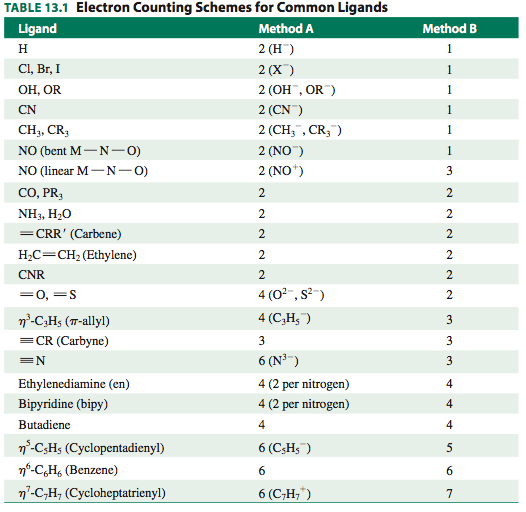
EXAMINING
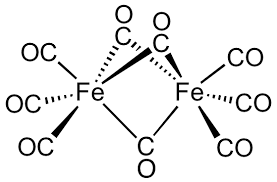
Since this compound is symmetric about the vertical mirror plane perpendicular to the screen (the plane perpendicular to the
In the case of
#:"C"-="O":#
It has a lone pair, and it is neutral, so it donates two electrons under either method. The
#6 xx 2 = bb12# electrons
to the effective atomic number.
Thus,
That means its effective atomic number is
#6 xx 2 + 26 = color(blue)bb(38)#

