A right triangle has sides A, B, and C. Side A is the hypotenuse and side B is also a side of a rectangle. Sides A, C, and the side of the rectangle adjacent to side B have lengths of #5 #, #2 #, and #4 #, respectively. What is the rectangle's area?

Question

2482 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-09-01T08:58:49

#A = 4 sqrt(21)#

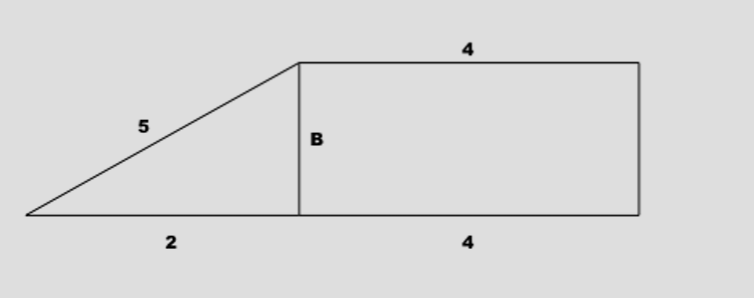
enter image source here

This is an image I created using the information you provided.

First, let's find the length of side #"B"# using Pythagoras' theorem:

#Rightarrow 5^(2) = "B"^(2) + 2^(2)#

#Rightarrow 25 = "B"^(2) + 4#

#Rightarrow 21 = "B"^(2)#

#therefore "B" = sqrt(21)#

Both of the widths of the rectangle should be labelled #"B"#, as they are equal.

The area of the rectangle will be the product of its length and width:

#Rightarrow A = "B" times 4#

#Rightarrow A = (sqrt(21)) times 4#

#therefore A = 4 sqrt(21)#

Therefore, the area of the rectangle is #4 sqrt(21)#.