Question

What does the mass of a black hole need to be in order for its mass divided by its volume to be equal to the density of water (1g/`cm^3`)?

Answer 1

`~7 xx 10^21` solar masses

Explanation:

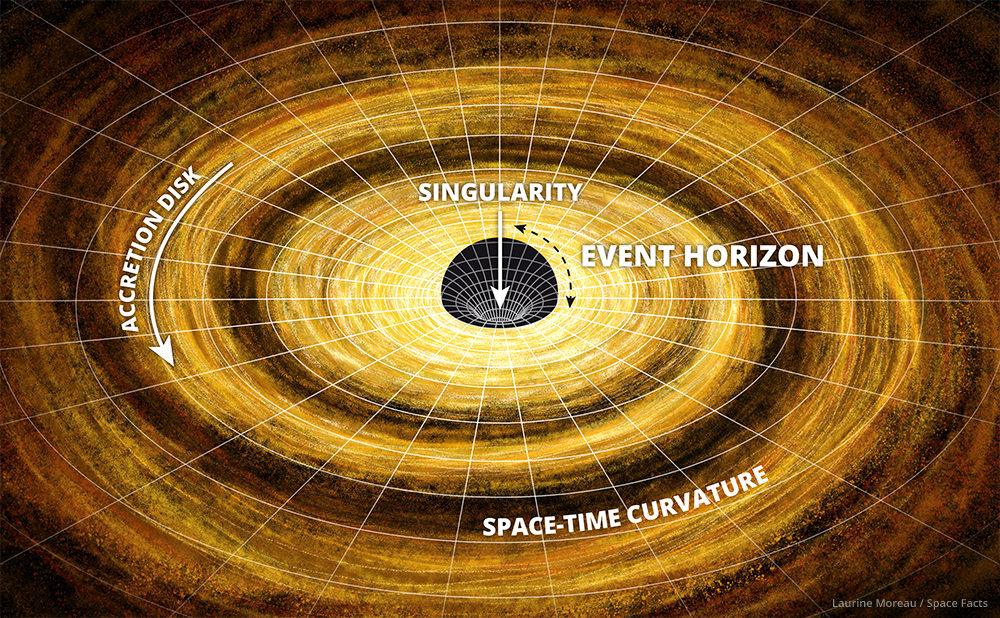
At its simplest, a black hole can be thought of as a collapsed star where all of the mass is concentrated into a single point in space, the singularity. Because it is a point, there is no volume. The density of the singularity is therefore infinite regardless of mass.

`"density" = "mass"/"volume" = "mass"/0 = oo`

That said, black holes have an event horizon, which is the point where light is "captured" by the black hole. If we treat this event horizon as a spherical boundary for the black hole, then we can use its volume for our density calculation instead of the singularity. Effectively, we are calculating the "average" density within the event horizon. The radius of the event horizon, called the Schwarzschild Radius, can be found using the following;

`R = (2MG)/c^2`

Where `M` is the mass of the singularity, `G` is the coefficient of gravity, and `c` is the speed of light in a vacuum. The volume of our spherical event horizon is therefore;

`V = pi R^2=4pi(MG)^2/c^4`

Our density formula from above is now much more interesting.

`rho = c^4/(4piMG^2)`

Or, with a little rearranging,

`M = c^4/(4pi rho G^2)`

Plugging in the constants and the density of water, `rho = 1 "g/cm"^2`, we can solve for our mass.

`M = (3xx10^10 "cm/s")^4/(4 pi (1 "g/cm"^2)(6.67 xx 10^-8 "cm"^3"/g/s"^2)^2) = 1.45 xx 10^55 g`

In more meaningful terms, this is equivalent to `~7 xx 10^21` solar masses, within the range of stellar black holes. I would like to reiterate that this is the average density for a black hole, and does not necessarily reflect the actual distribution of matter within the event horizon. A typical treatment of black holes effectively puts all of the mass in the infinitely dense singularity.