How do you write an equation of #y=sinx# with pi/2 units to the right and 3.5 units up?

1 Answer
Somebody N.
Nov 3, 2017

#y= sin(x-pi/2)+7/2#

Explanation:

Subtracting #pi/2# from #x# will move the graph #pi/2# units to the right. Adding #pi/2# to #x# will move the graph #pi/2# units to the left.

Adding a constant to #sinx# will translate the graph up for positive value and down for a negative value.

So:

#y=sinx# becomes #y= sin(x-pi/2)+7/2#

See graphs:

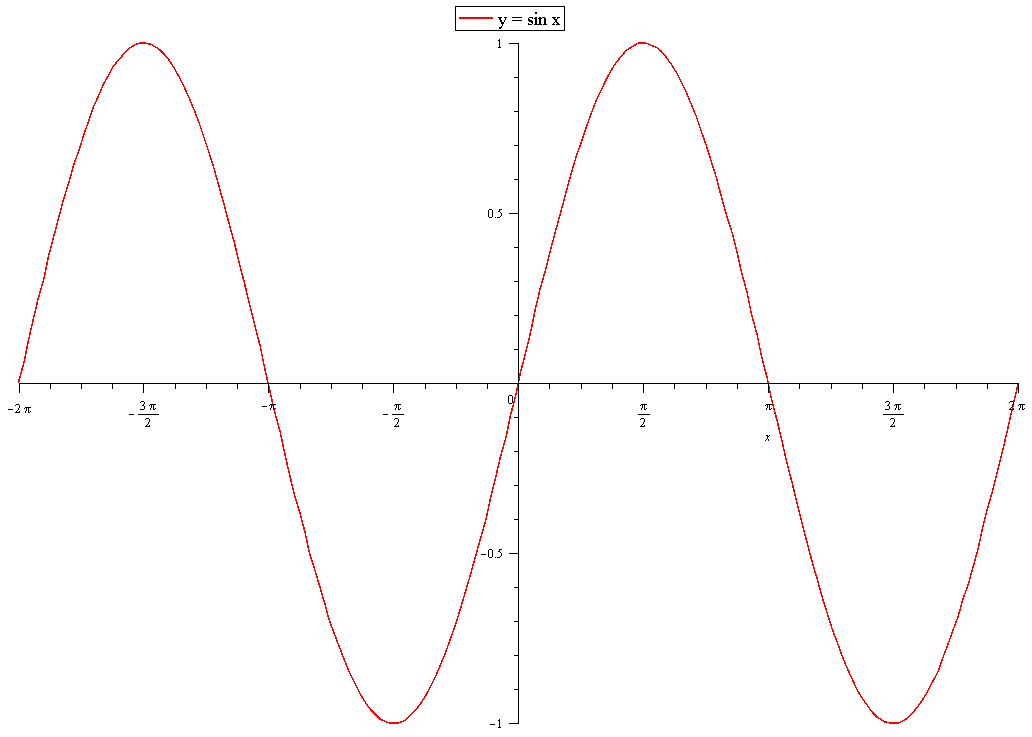
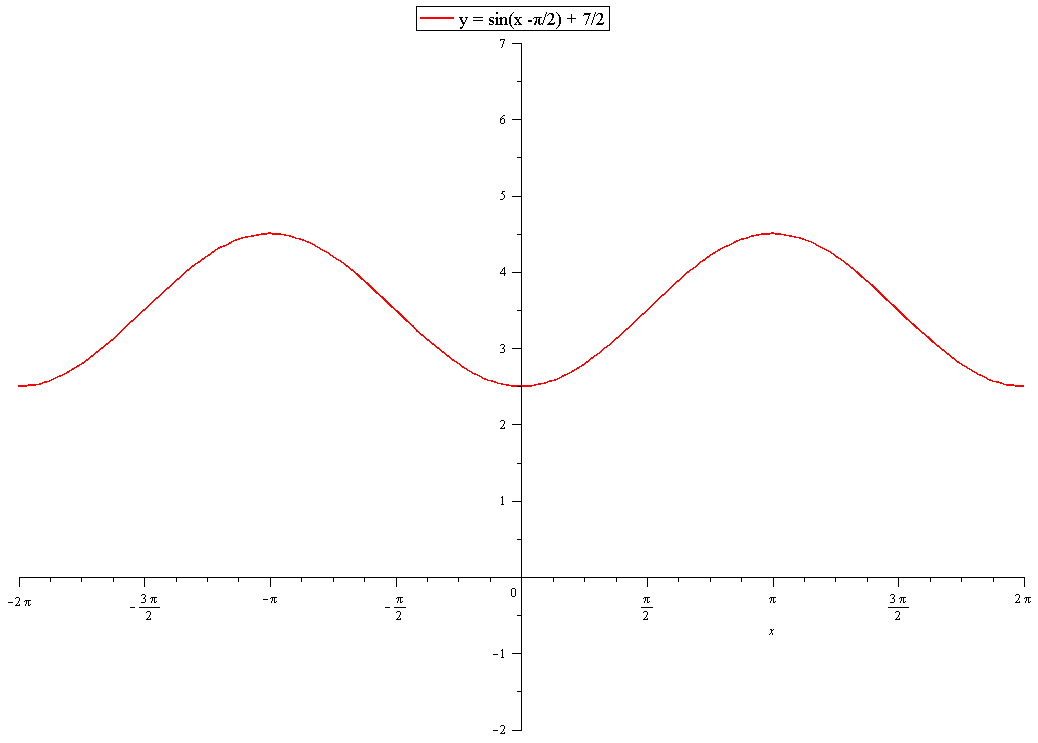
enter image source here

enter image source here

Related questions
See all questions in Translating Sine and Cosine Functions
Impact of this question
9094 views around the world
You can reuse this answer
Creative Commons License