How many quantum number sets can be written for an electron located in the #4d# subshell?
1 Answer
Here's what I got.
Explanation:
The first thing to note here is that the coefficient added in front of the name of the subshell tells you the energy shell in which the electron resides.
In your case, an electron located in the
#n = 4 -># the fourth energy shell
Now, the identity of the energy subshell is given by the angular momentum quantum number,
#l = 0 -># the#s# subshell#l = 1 -># the#p# subshell#l = 2 -># the#d# subshell
#vdots#
and so on. In your case, the electron is located in the
#l = 2#
Now, in order to describe an orbital, you need to have subscripts added to the identity of the energy subshell. The magnetic quantum number,
#m_l = {-2, - 1, 0, +1, +2}#
This means that the
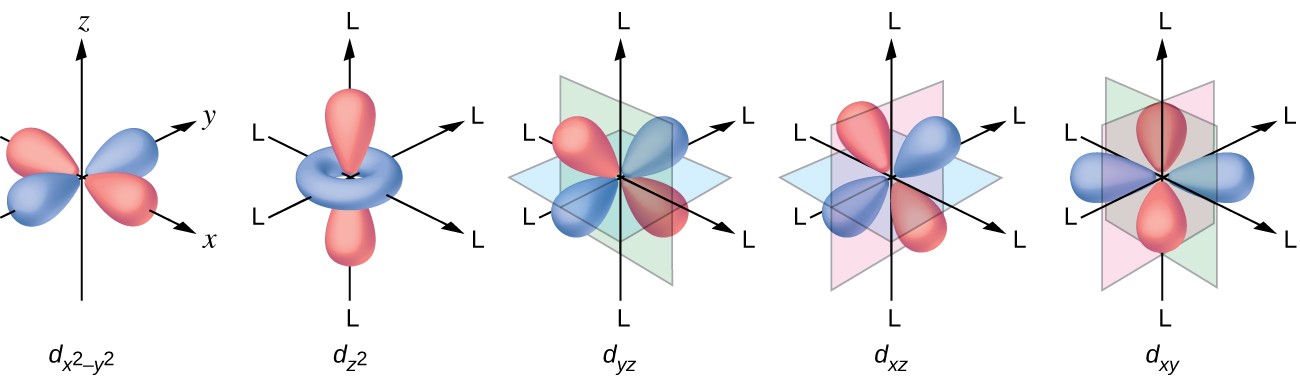
For example, you can have
#m_l = -2 -># the#4d_(x^2 - y^2)# orbital#m_l = -1 -># the#4d_(yz)# orbital#m_l = color(white)(+)0 -># the#4d_(z^2)# orbital#m_l = +1 -># the#4d_(xz)# orbital#m_l = +2 -># the#4d_(xy)# orbital
Finally, the spin quantum number,
#m_s = {+1/2, - 1/2}#
So, one set of quantum numbers that can describe an electron located in the $d# subshell would be
#n =4, l =2, m_l = 0, m_s = +1/2# This set describes an electron that is located on the fourth energy level, in the
#4d# subshell, in the#4d_(z^2)# orbital, and that has spin-up.
Another example would be
#n =4, l =2, m_l = +2, m_s = -1/2# This set describes an electron that is located on the fourth energy level, in the
#4d# subshell, in the#4d_(xy)# orbital, and that has spin-down.
Since the
You can find all

