Kite KLMN has vertices at K(1, 3), L(2,4), M(3,3), and N(2,0). After the kite is rotated, K' has coordinates (-3,1). How do you find the rotation, and include a rule in your description. Then find the coordinates of L', M',and N'?

1 Answer
sankarankalyanam
Feb 22, 2018

Rotated about origin by #(3pi)/2# clockwise.

#L’(-4,2), M’(-3,3)#

Explanation:

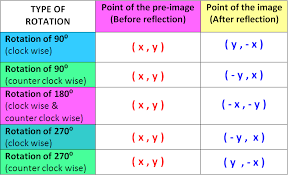
enter image source here

Given : #K (1,3), L (2,4), M(3,3), K’ (-3,1)#

#K(1,3) -> K’(-3,1)# changed from I quadrant to II quadrant.

I.e. rotated #(3pi)/2# clockwise.

That means, #((x),(y) )-> ((-y),(x))#

Hence, L((2),(4)) -> L’((-4),(2))#

#M ((3),(3)) -> M’((-3),(3))#

Related questions
See all questions in Rotations
Impact of this question
4087 views around the world
You can reuse this answer
Creative Commons License