What is the equation of the line that passes through #(-1,1) # and is perpendicular to the line that passes through the following points: #(-2,4),(-12,21) #?

Question

5361 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-03-11T10:56:56

#y=10/17x+27/17#

Consider the two points #(-2,4) and (-12,21)#

The straight line between them has the gradient:

#m=("change in "y)/("change in "x)# reading left to right on the x-axis

#m=(21-4)/(-12-(-2)) = -17/10#

The gradient of the perpendicular line is #-1/m =+10/17#

So we have #y=10/17x+c # passing through #(x,y)->(-1,1)#

So by substitution we get:

#y=10/17x+c color(white)("dddd")->color(white)("dddd")1=10/17(-1)+c#

#c=1+10/17 = 27/17#

#y=10/17x+27/17#

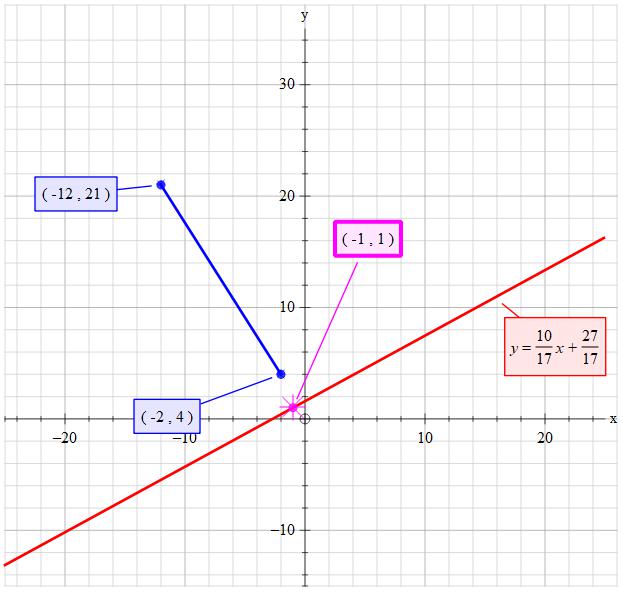
Tony B

Answer 2 · 2018-03-11T11:05:34

Equation of line is #10x-17y= -27#.

The slope of the line passing through #(-2,4) and (-12,21)#

is #m_1= (y_2-y_1)/(x_2-x_1)= (21-4)/(-12+2)= -17/10#

The product of slopes of the pependicular lines is #m_1*m_2=-1#

#:.m_2=-1/(-17/10)=10/17#. Hence the slope of the pependicular

line is #m_2=10/17# . Equation of line passing through #(-1,1)#

is #y-y_1=m_2(x-x_1) or y-1= 10/17(x+1)# or

# 17y-17= 10(x+1) or 10x-17y= -27#.

Equation of line is #10x-17y= -27#. [Ans]