How do you simplify #cos 2(pi/3)#?

Question

46674 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-03-19T05:59:27

The simplified answer is #-1/2#.

#color(white)=cos2(pi/3)#

#=cos(2*pi/3)#

#=cos((2pi)/3)#

You can use a unit circle to help you out:

enter image source here

We can see that #cos((2pi)/3)# is just #-1/2#.

Answer 2 · 2018-03-19T06:03:59

#cos 2 (pi/3) = 1/2#

let #theta = pi/3#

#cos 2 theta = cos 2(pi/3) = cos^2 (pi/3) - sin^(pi/3) color(white)(aaa)# as #cos (2 theta) = cos^2 theta - sin^2 theta# identity.

enter image source here
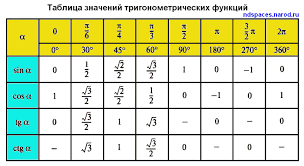
( useruploads.socratic.org )

#=> (sqrt3/2)^2 - (1/2)^2 = 3 / 4 - 1/4 = 1/2#