Which curve is more steeper, isothermal or adiabatic? Explain please
1 Answer
Adiabats are steeper than isotherms.
Explanation:
When we graph adiabatics and isotherms, we might do so on a P vs. V diagram, or a pressure vs. volume diagram. Therefore, the slope of any curve on the graph is
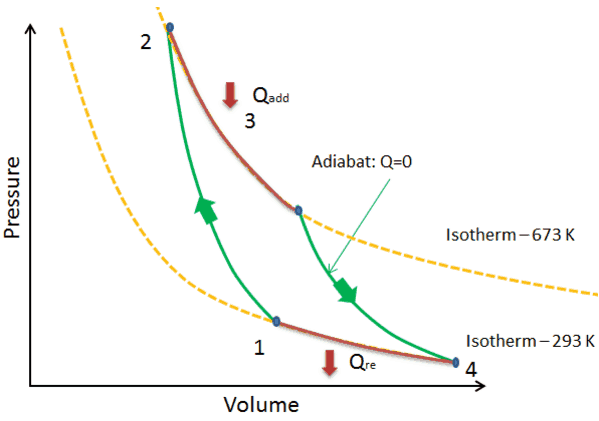 nuclear-power.net
nuclear-power.net
For an isothermal process, we know that there is no change in temperature, i.e.
PV="constant"
Let's say
=>P=c/V
Now we'll take the derivative to look at the change in the variables (derivative = slope):
=>(dP)/(dV)=-c/V^2
Let's rewrite our equation:
=>(dP)/(dV)=-c/V*1/V
From above
=>(dP)/(dV)=-P/V
For an adiabatic process, the energy input into the system by heating is necessarily zero, i.e.
=>PV^(gamma)=c
Again, we solve for
P=c/V^(gamma)
As with the isothermal process:
=>(dP)/(dV)=-gamma*c/(V^(gamma+1))
Where we have added one to
=>(dP)/(dV)=-gamma*c/V^(gamma)*1/V
=>(dP)/(dV)=-gamma*P/V
As gamma is always greater than 1, we see that the slope of an adiabat is greater than that of an isotherm by a factor of

