How do you know when to use the ambiguous case when finding possible lengths of triangles?
1 Answer
Mar 29, 2018
As listed below.
Explanation:
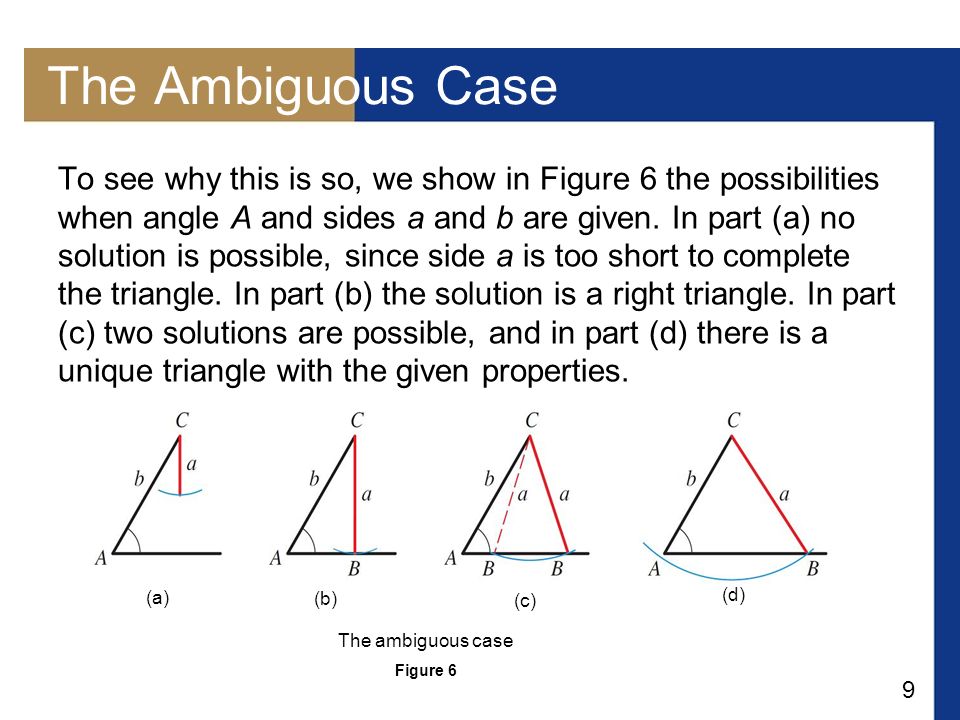
For those of you who need a reminder, the ambiguous case occurs when one uses the law of sines to determine missing measures of a triangle when given two sides and an angle opposite one of those angles (SSA). ... If angle A is acute, and a = h, one possible triangle exists
-
If angle A is acute, and a < h, no such triangle exists.
-
If angle A is acute, and a = h, one possible triangle exists.
-
If angle A is acute, and a > b, one possible triangle exists.
-
If angle A is acute, and h < a < b, two possible triangles exist.
-
If angle A is obtuse, and a < b or a = b, no such triangle exists.
-
If angle A is obtuse, and a > b, one such triangle exists.