How to use the discriminant to find out how many real number roots an equation has for #8b^2 - 6b + 3 = 5b^2#?

Question

3495 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-04-16T01:44:31

#color(crimson)("The given equation has TWO REAL ROOTS"#

For a given quadratic equation in the standard form #ax^2 + bx + c#,

#"Discriminant " D = b^2 - 4ac#

#8b^2 - 6b + 3 = 5b^2#

#8b^2 - 5b^2 - 6b + 3 = 0, " making R H S = 0"#

#3b^2 - 6b + 3 = 0#

#:. D = b^2 - 4 a c = (-6)^2 - (4 * 3 * 3) = 36 - 24 = 12, color(green)(" which is " > 0 " (Positive)"#

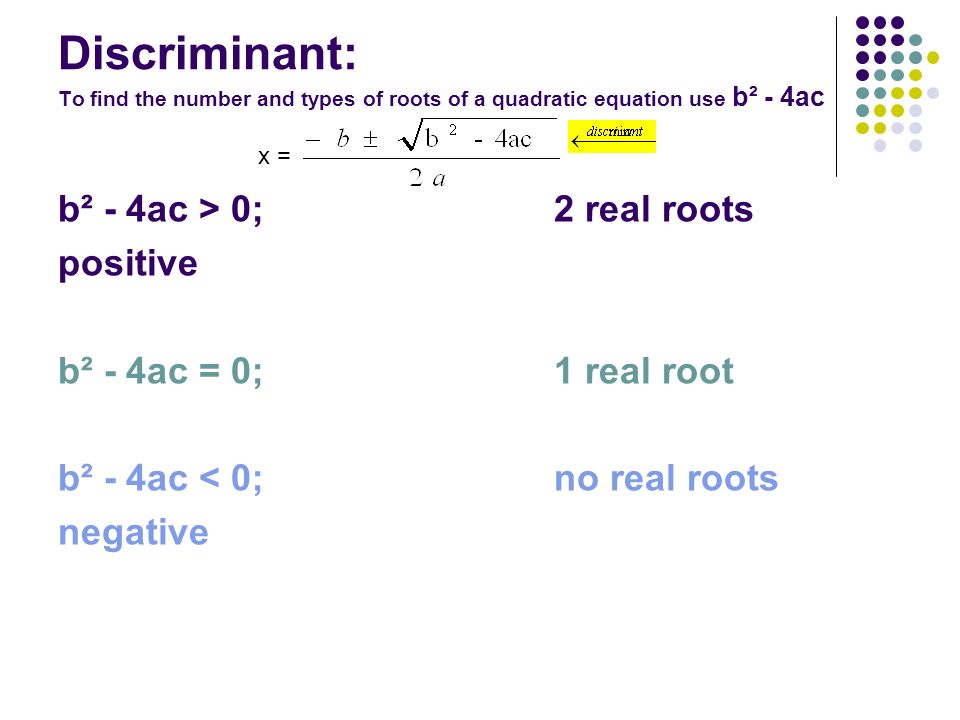
Hence #color(crimson)("The given equation has TWO REAL ROOTS"#