Question

Is a triangle with sides of 3,4,6 a right triangle?

Answer 1

It is not a right triangle.

Explanation:

To check if the sides are a right triangle, check if the sum of the squares of the two smaller sides equals the length of the square of the longest side.

In other words, check if it works with the Pythagorean theorem:

Does `3^2+4^2` equal `6^2`?

`3^2+4^2stackrel?=6^2`

`9+16stackrel?=36`

`25!=36`

Since `25` isn't `36` the triangle is not a right triangle.

Hope this helped!

Answer 2

A triangle with sides of `color(red)(3, 4 and 6` is `color(blue)(NOT` a Right triangle.

Explanation:

`" "`
We are given three sides of a triangle `3, 4 and 6`.

Pythagoras Theorem states that in a right angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.

To determine whether the three given sides form a right triangle, we use the Pythagoras Theorem to verify.

Draw a triangle, say `A, B, C` with the given magnitudes.

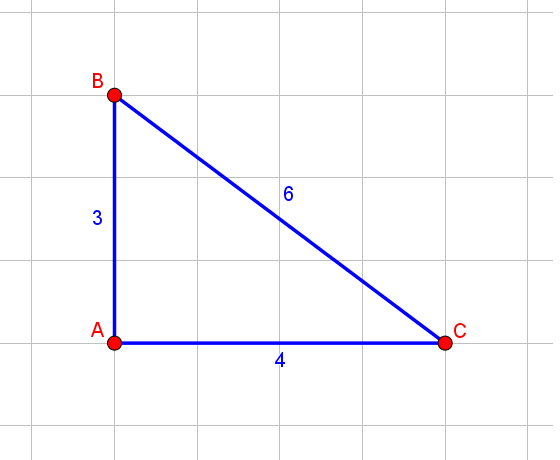
Note that the longest side (BC) has a magnitude of `6` units.

Hence, this must be the Hypotenuse, if triangle ABC is a right-triangle.

Does the angle `/_ CAB` make a right angle of `90^@`?

Verify that using the relationship between the hypotenuse and the other two legs of the triangle.

If `(AB)^2 + (AC)^2 = (BC)^2`, then we know that `BC` is the Hypotenuse and the triangle `ABC` is a right-triangle.

`bar (AB)=3`; `bar (AC) = 4`; and `bar (BC) = 6`

`(AB)^2 = 9`

`(AC)^2 = 16`

`(BC)^2 = 36`

`(AB)^2 + (AC)^2 = 9+16=25`

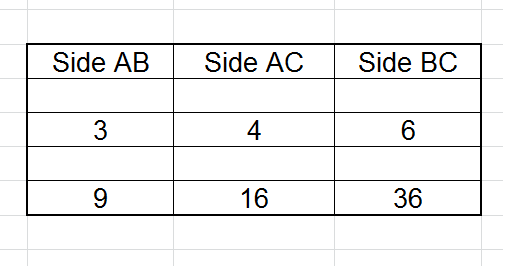
Hence,

`(AB)^2 + (AC)^2 != (BC)^2`

Hope it helps.