How to find the maximum distance traveled by a ball of mass M, tied to a light elastic string, projected vertically downwards with an initial velocity of Vo?
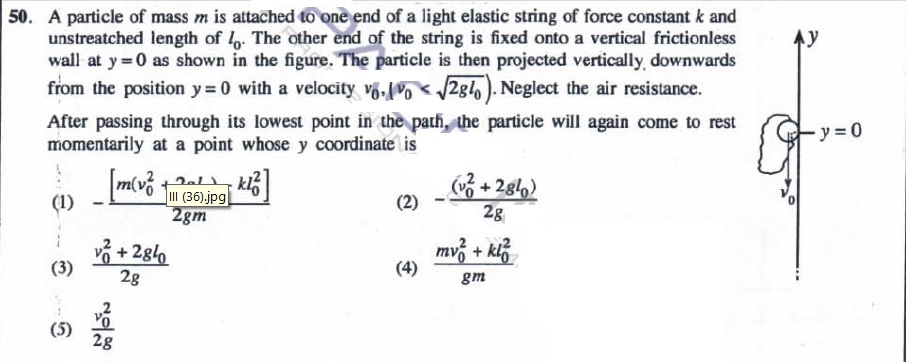
It is given that;
spring constant -k
Initial length - Lo
Vo < sqrt(2gLo)√2gLo

It is given that;
spring constant -k
Initial length - Lo
Vo <
2 Answers
Explanation:
Due to conservation of energy, the particle will pass via its starting point with upward velocity
Because:
v_o lt sqrt(2gl_o)vo<√2glo
Then:
1/2m v_o^2 lt m g l_o 12mv2o<mglo
ie the particle will stop before the string extends. All of its energy will be in the form of gravitational PE.
If it rises to height
(5)
Explanation:
The ball rises from the lowest point crosses
"Mechanical PE of string"+"GPE"="Initial KE"Mechanical PE of string+GPE=Initial KE
Inserting various values we get
"Mechanical PE of string"+mgy=1/2mv_0^2Mechanical PE of string+mgy=12mv20 ......(1)
It is given that
v_0" < "sqrt(2l_0g)v0 < √2l0g
=>"Initial KE"=1/2mv_0^2" < "1/2mxx2l_0g⇒Initial KE=12mv20 < 12m×2l0g
=>"Initial KE < "mgl_0⇒Initial KE < mgl0
=>"Initial KE"⇒Initial KE is not sufficient to raise the ball from the mean positiony=0y=0 to its unstreched lengthl_0 on its way up.
As the string remains slack, its mechanical PE
mgy=1/2mv_0^2
=>y=v_0^2/(2g)

