Question

How do you use the distributive property to simplify `–9 + 4(2x – 1) + 8`?

Answer 1

`8x - 5`

Explanation:

`-9 + 4(2x-1) + 8`

First, we know that `-9 + 8` simplifies to `-1`, so it becomes;
`-1 + 4(2x-1)`

Now we use the distributive property (shown below) to simplify/expand `4(2x-1)`:

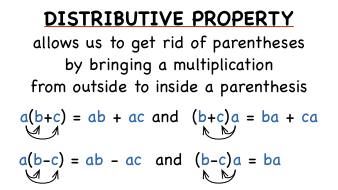
Following this image, we know that:
`color(blue)(4(2x-1) = (4 * 2x) + (4 * -1) = 8x - 4)`

Put that back into the expression:
`-1 + 8x - 4`

Simplify `-1 - 4 = -5`:
Therefore, the simplified expression is:
`-5 + 8x`

We typically put variables before pure numbers, so the expression becomes:
`8x - 5`

Hope this helps!