Question

How do you graph two complete cycles of `y=1/2sint`?

Answer 1

`" "`
Please read the explanation.

Explanation:

`" "`
Create a data table for the Parent function `color(red)(y=f(t)=sin(t)` as shown below:

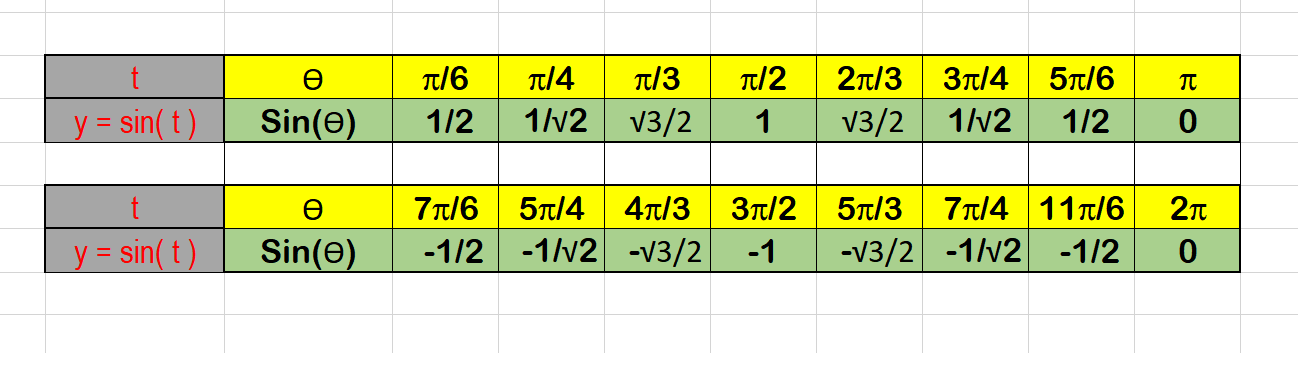
Graph the Parent function as shown below:

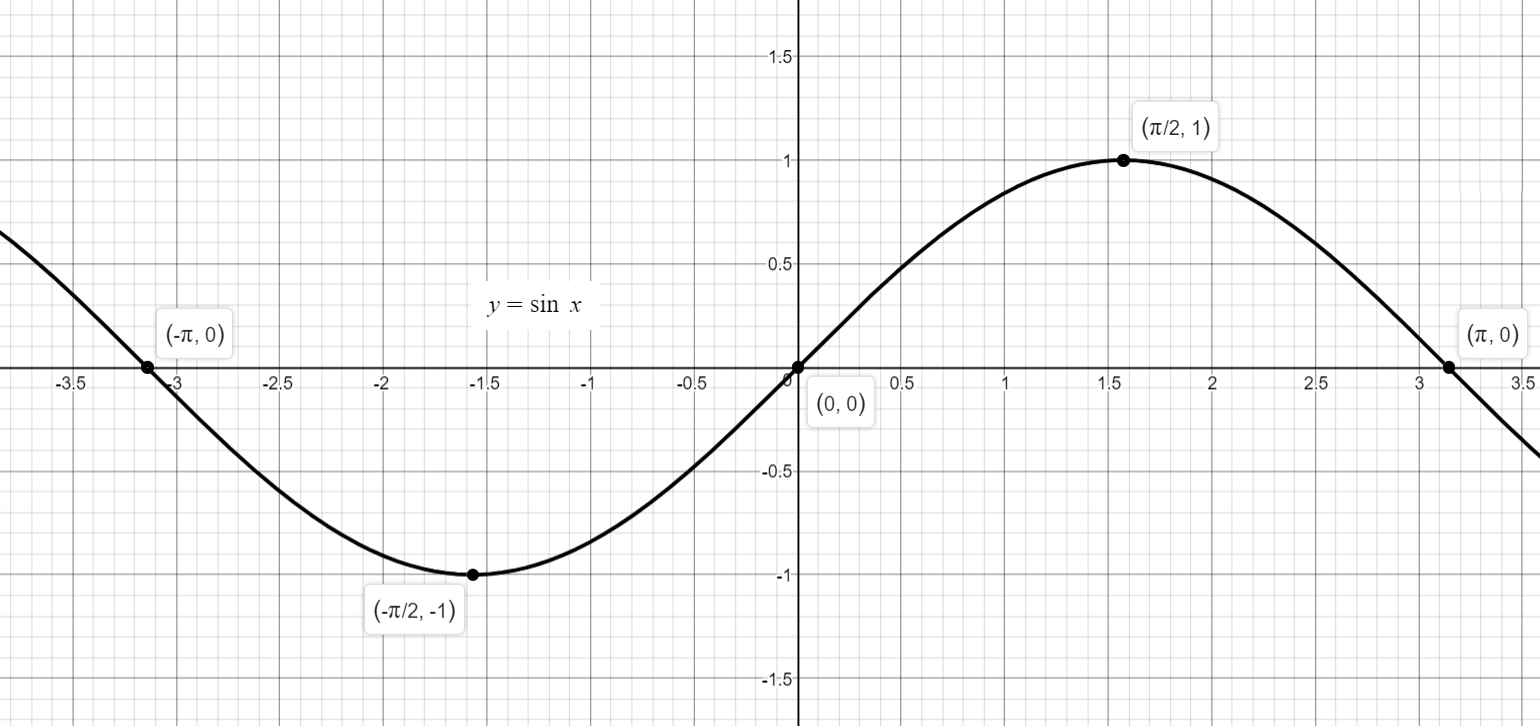
Next, graph the function: `color(red)(y=f(t)=(1/2)*sin(t)`

Now, we understand the behavior of the graph

`color(red)(y=f(t)=(1/2)*sin(t)`

by comparing it to the parent graph.

The question reads draw two complete cycles.

The Period of a trigonometric function is the horizontal length of one complete cycle.

The Sine curve, `color(red)(y=sin(t)`, has a Period of `color(blue)(2 pi`.

The Sine curve, `color(red)(y=(1/2)*sin(t)`, has also a Period of `color(blue)(2 pi`.

We need to restrict or place a constraint using an appropriate condition as shown below:

Again, we can compare the restricted graphs of both the parent function and the given function for easy comprehension.

I hope it helps.