Question

Which `d` orbital is specified by `Y(theta,phi) = (5/(8pi))^(1//2) (3cos^2theta - 1)`?

Answer 1

Your angular wave function looks off. Every wave function `psi_(nlm_l)`, which describes the state of a quantum mechanical system, must be at LEAST

• orthogonal (`int_"allspace" psi_i^"*"psi_jd tau = 0` where `i ne j`)
• normalizable (`int_"allspace" psi_i^"*"psi_id tau = 1`)

and yours is not. The correct `Y_(l)^(m_l)(theta,phi)` is:

`color(blue)(Y_(2)^(0)(theta,phi) = (5/(16pi))^"1/2" (3cos^2theta - 1))`

In this case, what we have here is ONLY the angular component of the wave function for the `d_(z^2)` orbital using spherical coordinates. Actually, without knowing the radial component, we do not know the principal quantum number of your `d_(z^2)` orbital.

The full wave function in spherical harmonics is written as the product of the radial and angular components:

`\mathbf(psi_(nlm_l)(vecr,theta,phi) = R_(nl)(vecr)Y_(l)^(m_l)(theta,phi))`

For instance, the full wave function for the `3d_(z^2)` orbital is:

`psi_(3d_(z^2)) = psi_(320)(vecr,theta,phi) = R_(32)(vecr)Y_(2)^(0)(theta,phi)`

`= 1/(81sqrt(6pi))(Z/(a_0))^"3/2" ((Zr)/(a_0))^2e^(-Zr"/"3a_0) (3cos^2theta - 1),`

where `Z` is the atomic number and `a_0` is the Bohr radius (`5.29177xx10^(-11) "m"`).

This, you would see is quite different and much more complicated.

You did not include `R_(nl)(vecr)`, so the best I can do is say that you have accidentally specified all `d_(z^2)` orbitals in existence. There exist the `3d_(z^2)`, `4d_(z^2)`, `5d_(z^2)`, and `6d_(z^2)` thus far that have been observed.

Generally they look like dumbbells along the z-axis with a torus (donut) on the xy-plane. The torus has the OPPOSITE sign to the lobes, and the lobes have the SAME sign as each other.

The `d_(z^2)` have conic nodal surfaces. Specifically:

• The `3d_(z^2)` has `n - l - 1 = 3 - 2 - 1 = 0` radial nodes and `n - 1 = 2` total nodes. Thus, it has two angular nodes.
• The `4d_(z^2)` has `n - l - 1 = 4 - 2 - 1 = 1` radial node and `n - 1 = 3` total nodes. Thus, it has two angular nodes as before.
• The `5d_(z^2)` has `n - l - 1 = 5 - 2 - 1 = 2` radial nodes and `n - 1 = 4` total nodes. Thus, it has two angular nodes as before.
• The `6d_(z^2)` has `n - l - 1 = 6 - 2 - 1 = 3` radial nodes and `n - 1 = 5` total nodes. Thus, it has two angular nodes as before.

So you can see the pattern that the `d_(z^2)` orbital simply gains radial nodes as the quantum number `n` increases.

For instance, here is a picture of the `5d_(z^2)` orbital:

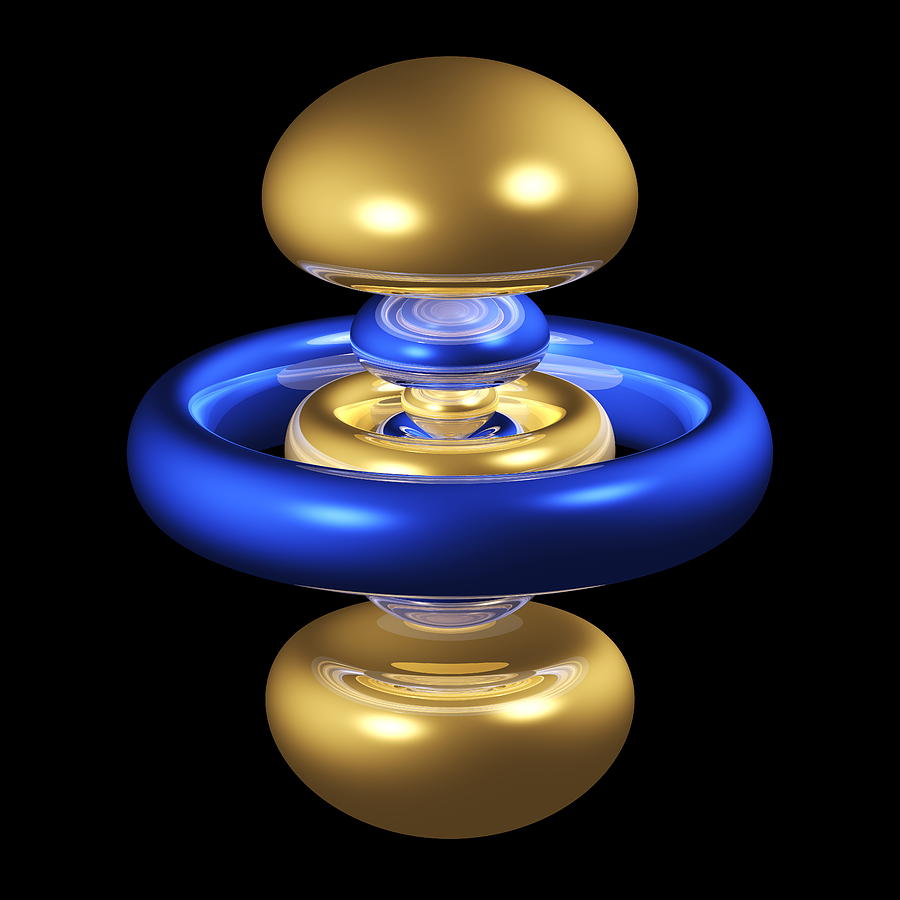
Now compare that to the `3d_(z^2)` orbital.

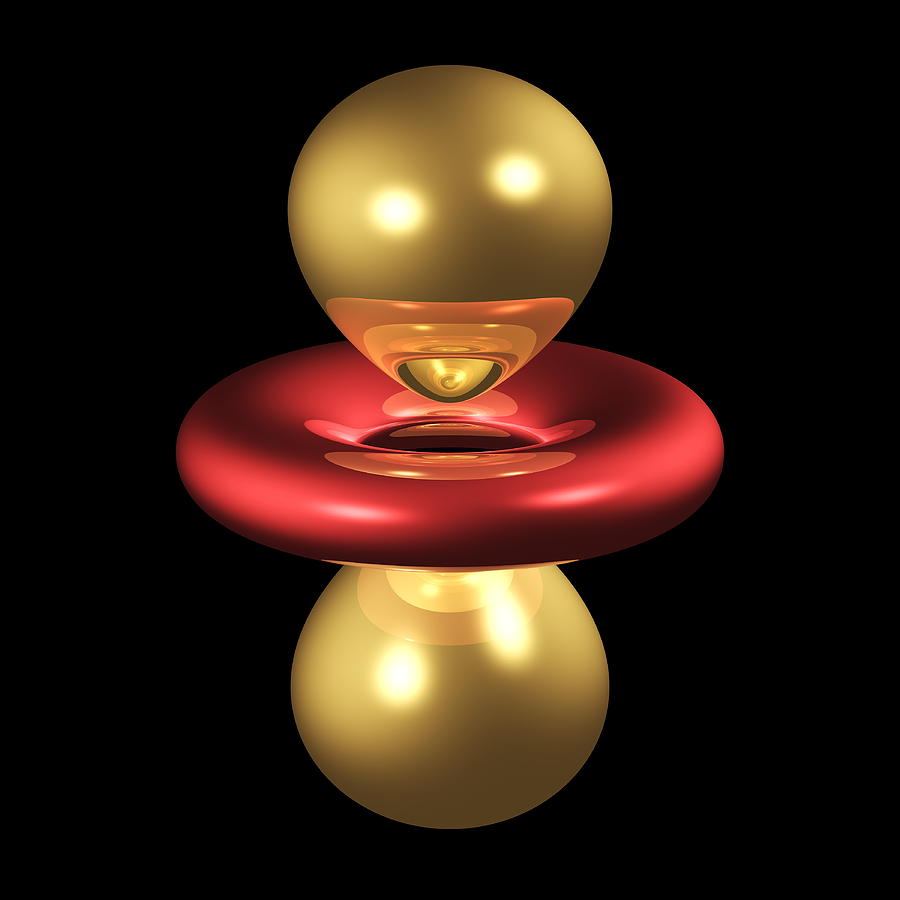
You should notice that the `5d_(z^2)` is obviously more complex at the torus.