Question

Which of the following is NOT a valid quantum number set for an orbital?

Is `A` wrong?

`A)` `(n,l,m_l) = (3,-3, 2)`
`B)` `(n,l,m_l) = (4, 3, 0)`
`C)` `(n,l,m_l) = (2, 1, 1)`
`D)` `(n,l,m_l) = (3, 2, 0)`

Answer 1

Recall the following relevant rules:

1. The value of the angular momentum quantum number `l` can at most be `n - 1`, one less than the principal quantum number `n`. Also, `l = 0, 1, 2, . . .`, so `l >= 0`.
2. `m_l` can only be in the set `{-l, -l+1, . . . , 0, . . . , l-1, l}`. That is, the magnitude of any given `m_l` can at most be `l`.

In fact, you are correct that it is `A` that does NOT occur. `n = 3` specifies all atomic orbitals at the third energy level (`3s, 3p, 3d`), while `l = 3`, an `f` orbital, is only possible at `n = 4` or above (for example, `4f, 5f, . . .` orbitals). Furthermore, `l >= 0`, so the value of `l = -3` isn't even valid.

`B`, `C`, and `D` are all valid configurations, which are not the incorrect one you are looking to eliminate.

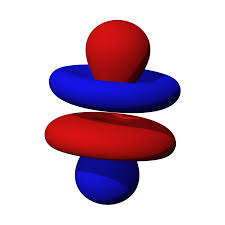
• `B` specifies a `4f` orbital of `m_l = 0`, namely the `4f_(z^3)`.

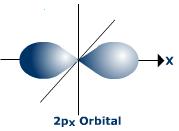
• `C` specifies a `2p` orbital of `m_l = +1`, such as the `2p_x`.

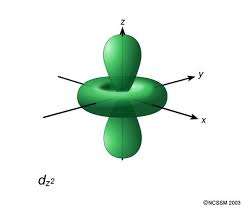
• `D` specifies a `3d` orbital of `m_l = 0`, namely the `3d_(z^2)`.