A box with an initial speed of 5 m/s5ms is moving up a ramp. The ramp has a kinetic friction coefficient of 3/7 37 and an incline of (3 pi )/8 3π8. How far along the ramp will the box go?
1 Answer
Apr 23, 2017
The distance is
Explanation:
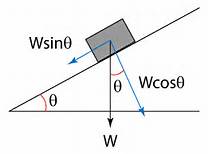
Solving in the direction of the plane
The component of the weight is
Applying Newton' second Law
Therefore,
The initial velocity is
We apply the equation of motion

