Question

A truck pulls boxes up an incline plane. The truck can exert a maximum force of `4,800 N`. If the plane's incline is `(3 pi )/8` and the coefficient of friction is `9/8`, what is the maximum mass that can be pulled up at one time?

Answer 1

The greatest mass that can be pulled (at constant speed) up the incline is 249.5 kg.

Explanation:

There are three forces that must be considered in this problem: gravity, friction and an applied force. Since we are looking for the maximum mass that can be pulled up the incline, we must consider this a case of zero acceleration, and our three forces must balance (as vectors, they add to zero).

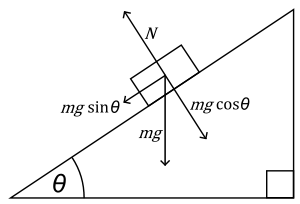
Next, since this is a two-dimensional problem, we must consider force components and resolve the forces into these components. The technique involves creating perpendicular components such that one set will act paralle to the incline (and the other will be perpendicular to it, of course).

First, the applied force: This force is entirely parallel (up along the plane); it has no perpendicular component, so

`F_(a||) = 4800 N`

Next, gravity. From the diagram:

`F_(g||) = mgsintheta = mgsin((3pi)/8)`

`F_(g_|_) = mgcostheta = mgcos((3pi)/8)`

Finally, friction. This also points parallel to the plane (but down the plane):

`F_f=mumgcostheta=mumgcos((3pi)/8)`

To finish the problem, we require that the three parallel components total zero:

`F_(||)=4800 N - mgsin((3pi)/8) - mumgcos((3pi)/8) = 0`

(Note that the negative signs are used for the direction "down the plane".)

Solving, by inserting the values we are given:

`4800 = m(9.8)(0.924)+(9/8)m(9.8)(0.924)`

Factor `m` from the two terms on the right:

`4800=m(9.054+10.187)`

`m=(4800)/(19.241) = 249.5 kg`