Question

An object with a mass of `10 kg` is on a plane with an incline of `- pi/4`. If it takes `12 N` to start pushing the object down the plane and `6 N` to keep pushing it, what are the coefficients of static and kinetic friction?

Answer 1

`mu_s = 2.70`

`mu_k = 1.85`

Explanation:

We're asked to find the coefficients of static friction `mu_s` and kinetic friction `mu_k`.

NOTE: Since the given angle is negative (`-pi/4`), I'll assume this is the angle of depression (the topmost angle in the above image), which means the angle if inclination is

`pi/2 - pi/4 = ul(pi/4`

I will use this angle for the problem's work.

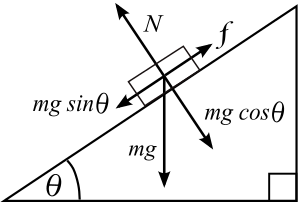
The coefficient of static friction `mu_s` is given by the equation

`f_s = mu_sn`

where

• `f_s` is the magnitude of the static friction force (the maximum allowed force before the object begins to move)

• `n` is the magnitude of the normal force exerted by the incline plane (equal to `mgcostheta`)

We realize that the object starts to move when the static friction force is equal in magnitude to the other forces acting on the object (gravitational and applied force).

Thus,

`f_s = mgsintheta + F_"applied"`

We're given the mass is `10` `"kg"`, the angle of inclination is `pi/4`, and that the applied necessary force is `12` `"N"`, so we have

`f_s = (10color(white)(l)"kg")sin(pi/4) + 12` `"N"` `= 19.1` `"N"`

The magntide of the normal force `n` is

`n = mgcostheta = (10color(white)(l)"kg")cos(pi/4) = 7.07` `"N"`

Thus,

`mu_s = (f_s)/n = (19.1cancel("N"))/(7.07cancel("N")) = color(red)(ul(2.70`

The coefficient of static friction `mu_k` is given by

`f_k = mu_kn` (notice the similarities to static friction)

where `f_k` is the magnitude of the kinetic friction force (the retarding force acting while the object is in motion).

The magnitude of the kinetic friction force will be equal to the applied pushing force (`6` `"N"`) plus the gravitational force (equal to `mgsintheta`):

`f_k = 6` `"N"` `+ 7.07` `"N"` `= 13.1` `"N"`

Therefore, we have

`mu_k = (f_k)/n = (13.1cancel("N"))/(7.07cancel("N")) = color(blue)(ul(1.85`