Question

An object with a mass of `8 kg` is on a ramp at an incline of `pi/8`. If the object is being pushed up the ramp with a force of `9 N`, what is the minimum coefficient of static friction needed for the object to remain put?

Answer 1

`mu_s >= 0.290`

Explanation:

We're asked to find the minimum value of the coefficient of static friction `mu_s` between the incline and object so that the object remains stationary.

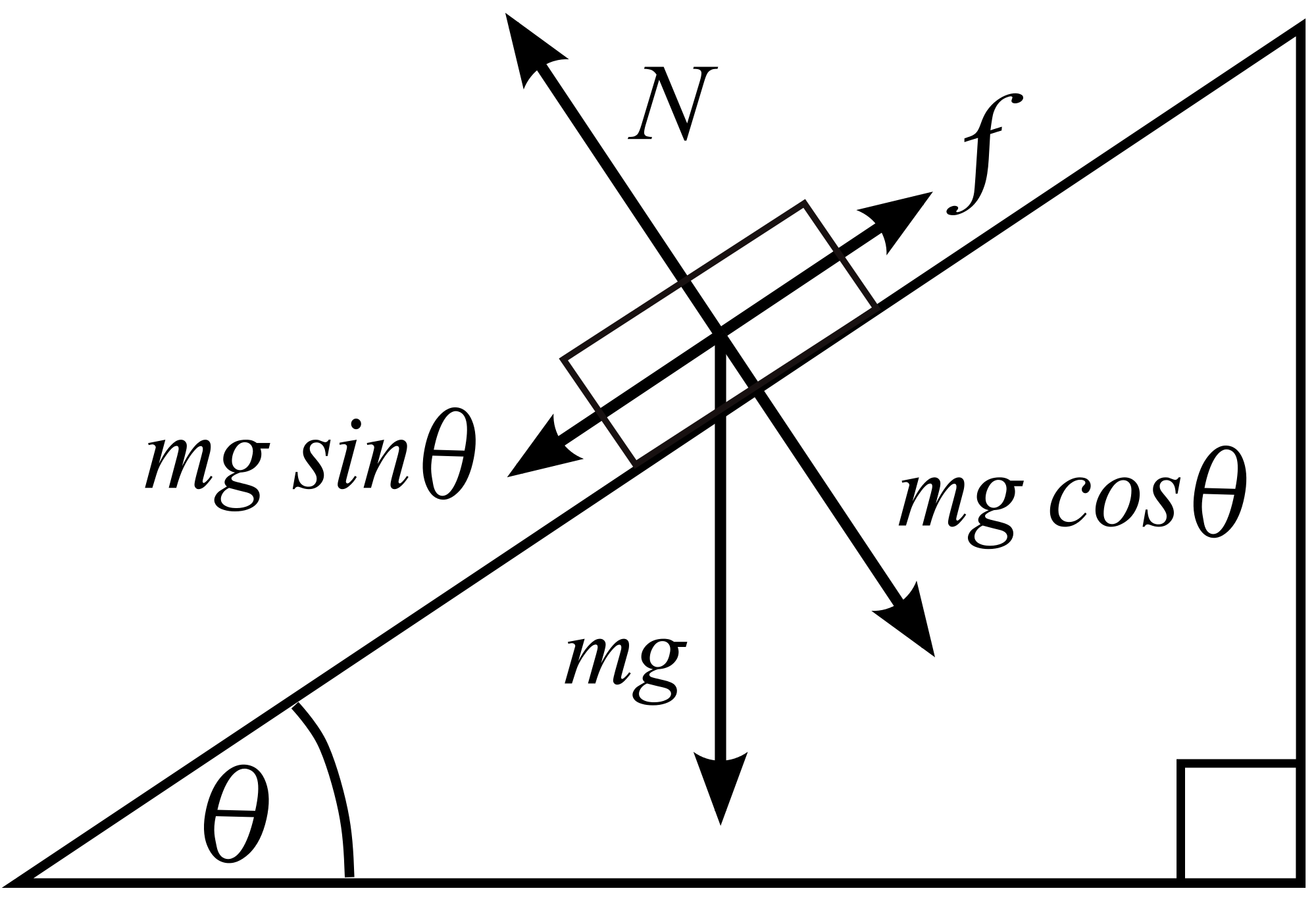
Since its mass is given as `8` `"kg"`,

`mgsintheta = (8color(white)(l)"kg")(9.81color(white)(l)"m/s"^2)sin(pi/8) = 30.0` `"N"`

The normal force magnitude `n` is

`n = mgcostheta = (8color(white)(l)"kg")(9.81color(white)(l)"m/s"^2)cos(pi/8) = 72.5` `"N"`

Since the object is supposed to be stationary, the body is in equilibrium (sum of all forces acting on body equals zero), so (taking positive `x` direction as up the incline)

`sumF_x = F_"applied" + f_s - mgsintheta = 0`

So

`f_s = mgsintheta - F_"applied"`

The applied force is given as `9` `"N"`, so we have

`f_s = 30.0` `"N"` `- 9` `"N"` = `21.0` `"N"`

Now that we know the static friction force `f_s`, we can figure out the coefficient of static friction using the equation

`f_s <= mu_sn`

`mu_s >= (f_s)/n = (21.0color(white)(l)"N")/(72.5color(white)(l)"N") = color(red)(0.290`